CHAPTER 24
LIQUID METAL FUEL REACTOR DESIGN STUDY¹⁰⁵

24-1.Comparison Of Two-Fluid And Single-Fluid
LMFR Designs

In Chapter 18, the two-fluid and the single-fluid externally cooled LMFR concepts were discussed in a general way. It was pointed out that the two-fluid design has the better breeding possibilities but is somewhat more complex than the single-fluid reactor. In this chapter a complete design study of a two-fluid full-sized LMFR reactor is described and discussed, and a shorter discussion of a single-fluid design study follows. This does not mean that one design is necessarily favored over the other. In fact both of these designs are being studied very extensively.

24-2. Two-Fluid Reactor Design

24-2.1 General description.

The two-fluid externally cooled LMFR concept consists of a relatively small core surrounded, for the most part, by a blanket containing fertile material. The core is composed of high-density, impervious graphite through which vertical channels are drilled to allow circulation of the fuel coolant. The fuel in the core is dissolved U²³³ or U²³³ dissolved and suspended in liquid bismuth. The fluid fuel also acts as coolant for the core system. The required coolant to moderator ratio is obtained by proper size and spacing of the fuel coolant channels.

The blanket is constructed of high-density graphite through which flows a liquid bismuth slurry containing the bred U²³³ fuel and thorium, the fertile material. In this study, thorium is assumed to be suspended in bismuth as thorium bismuthide, although thorium oxide particles could be used. The blanket is wrapped around the core as completely as possible for good neutron economy. An important economic consideration is the degree of end blanketing which can be achieved while keeping coolant velocities below the allowable limit. Several blanket designs were investigated, but a complete study for obtaining the best end blanket design has not yet been carried out.

24-2.2 General specifications.

Unless otherwise noted, the specifications listed below are common to all calculations performed in this design.

Total power	825 mw (thermal) 315,000 kw (electrical)
Coolant to moderator ratio in core, VBi/VC	1.22
Coolant to moderator ratio in blanket, Vslurry/VC	0.50
Core-blanket barrier material	graphite
Blanket thickness	3.0 ft
Blanket slurry composition:
Bismuth	90w/o
Thorium, as Th3Bi5	10w/o
Coolant inlet temperature	750°F
Coolant outlet temperature	1050°F

 

Nuclear calculations utilizing latest cross sections and multigroup diffusion theory indicate that the values 1.22 and 0.50 listed above are close to the optimum.

The several factors which dictated the choice of a bismuth-to-carbon volume ratio merit some attention. There are some losses of neutrons due to capture in graphite. Hence, one would wish to use only enough graphite to sufficiently thermalize the reactor. If too little graphite is used, the critical mass will be large. It is suspected that the 7/ value for U²³³ may be lower in the epithermal than in the thermal energy range. This would make it desirable to keep the reactor thermal. It was found that bismuthto-carbon volume ratios in the range of 0.5 to 2.0 satisfy these various requirements quite well. It may be further observed by referring to Fig. 24-1 that breeding improves with an increase in the bismuth-to-carbon volume ratio. However, the maximum bismuth-to-carbon volume ratio acceptable on the basis of structural limitations was 1.22, and consequently this core diameter is 155.7 cm (61 in.) at a bismuth-to-carbon volume ratio of 1.22, assuming a cylinder with its height equal to diameter.

Blanket slurry-to-graphite volume ratio and blanket thickness. A series of calculations were made to estimate the most economical parameter values for the blanket. Blanket slurry-to-graphite volume ratio and blanket thickness were varied to give the best breeding ratio consistent with reasonable bismuth holdup. Figures 24-2 and 24-3 demonstrate the effects of varying blanket composition and thickness on breeding ratio. The slurry-to-graphite volume ratio was set at 0.5 and the blanket thickness was set at 3.0 ft.

Study of design parameters. The parameters investigated in the following analysis are (1) end blanket design, (2) power fraction in the blanket, and (3) fission product poison level in the core.

 



FIG. 24-1. Breeding gain vs. bismuth-to-carbon volume ratio in core.

 



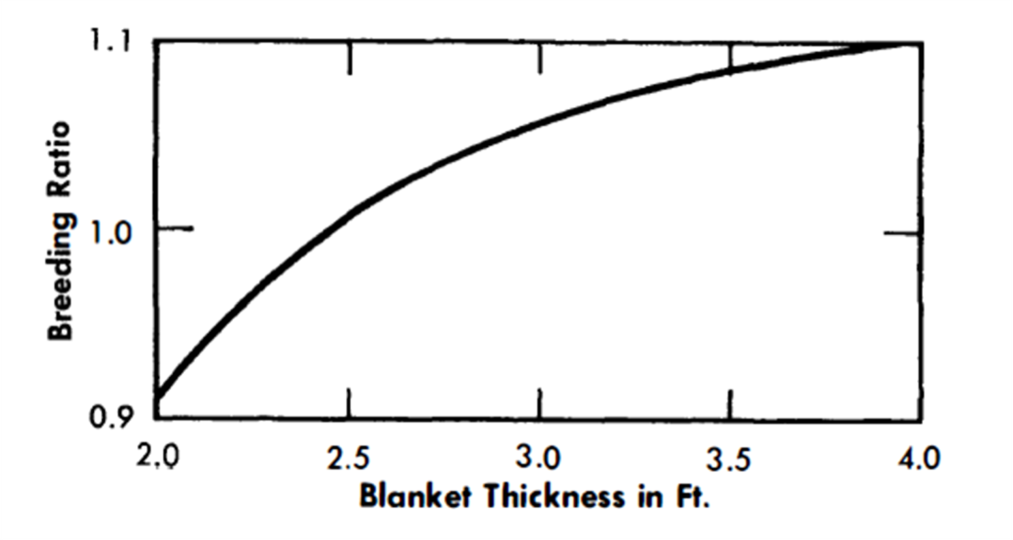
FIG. 24-2. Breeding vs. blanket thickness for slurry-to-carbon volume ratio = 1.00 and bismuth to carbon volume ratio in core =1.00.



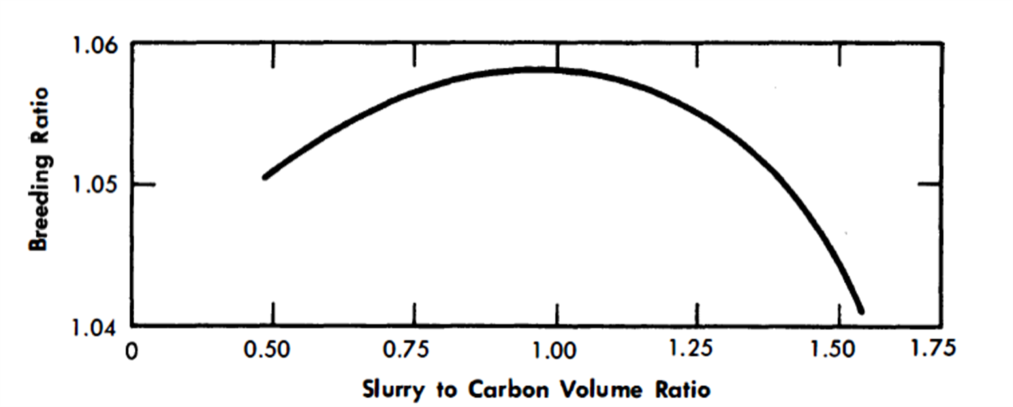
FIG. 24.3. Breeding vs. slurry-to-carbon volume ratio in blanket for bismuth to carbon volume ratio = 1.00 and blanket thickness = 3 ft.

24-2.3 End blanket effects.

A series of nuclear calculations were performed to determine the effects of end blanket design upon breeding ratio and critical fuel concentration. Two extreme blanket designs were considered. In the most optimistic case, a spherical core, equivalent to a 61-in.diameter cylinder, was surrounded by a 3-ft spherical blanket. The pessimistic calculations assumed a cylindrical core with a diameter of 61 in., height equal to 1.5 times the diameter, a 3-ft radial blanket, and no end blanket. Critical values of fuel concentrations and breeding ratio were calculated for four power fractions in the blanket for each design.

 

Table 24-1
Criticality Calculations For Two-Fluid LMFR
With And Without End Blankets

Case	N23/NBi x 106	Ratio of blanket power to total power	Breeding ratio	Blanket thickness, ft	Geometry
Core
Blanket
I	559	152	0.0665	1.053	3.0	Full Blanket
II	530	534	0.205	1.051	3.0	”
III	461	1600	0.445	1.039	3.0	”
IV	436	2100	0.515	1.033	3.0	”
V	403	1050	0.272	0.80	3.0	No end Blanket
VI	366	2100	0.425	0.82	3.0	”
VII	347	2808	0.492	0.83	3.0	”
VIII	403	1050	0.272	-	4.0	”

 

 

All calculations were performed for hot, clean conditions with an average temperature of 900°F. A two-group, multiregion code was used to solve the diffusion equations, and a 37-group spectral code was used to determine the two-group nuclear constants. The results of these calculations are tabulated in Table 24-1. The breeding ratio is decreased 0.20 to 0.25 by completely eliminating the end blankets. This is due primarily to the added neutron leakage out the ends of the core, despite the fact that the core height is increased. Although the critical mass of fuel in the core is higher without end blankets, the fuel concentration is somewhat lower due to the increased core volume.

 



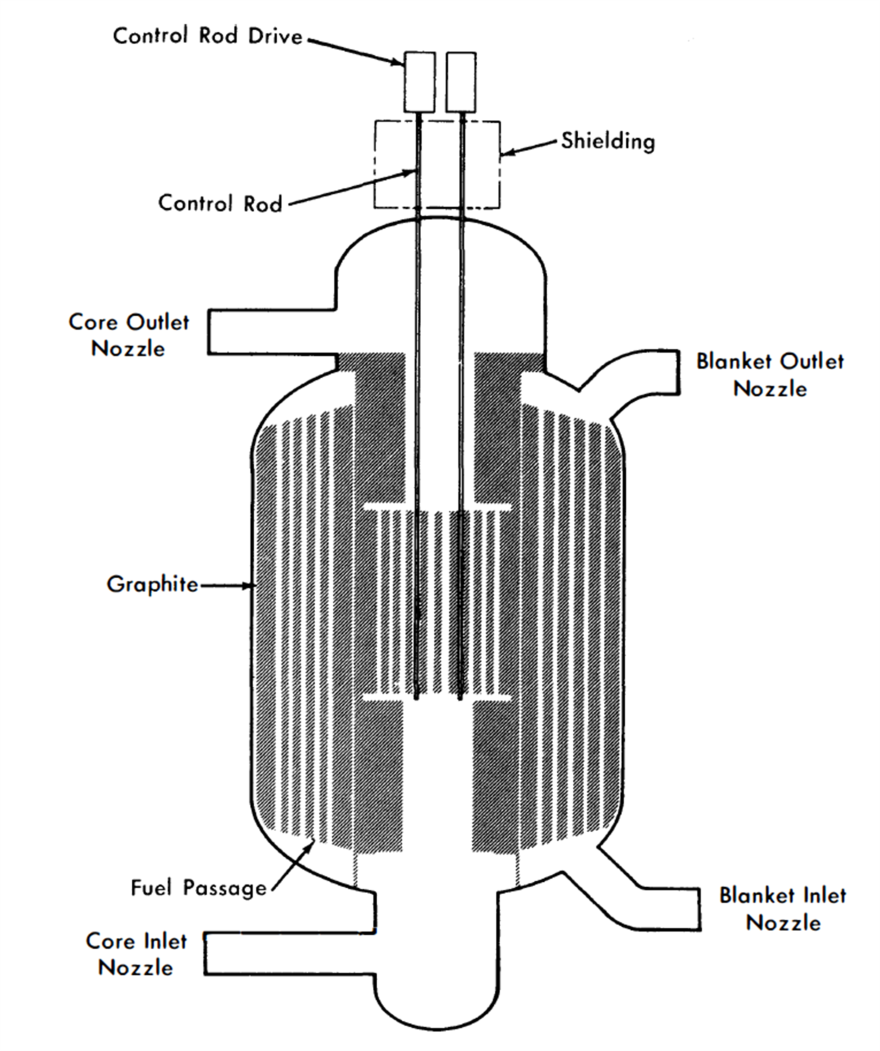
FIG. 24-4. Two-region, externally cooled liquid metal fuel reactor.

 

 

The actual core and blanket design is between the two extremes assumed in these calculations. The blanket can be extended beyond the end boundaries of the core, and a graphite reflector can cover the ends of the core except for the coolant inlet and outlet. Cooling becomes a serious design problem, if the end reflector is replaced with blanket material. The design in Fig. 24-4 is a substantial improvement over no end blanket or reflector. However, further improvement in breeding ratio could be achieved with even better end blanket designs.

24-2.4 Power level in the blanket.

For a given geometry, coolant-to-moderator ratio, and thorium concentration in the blanket, specification of the fraction of total fissions generated in the blanket establishes a unique set of values for fuel concentration in the blanket, fuel concentration in the core, and fissions generated in the core. For simplicity, the power generated in a region is assumed directly proportional to the fissions in that region. The data in Table 24-1 indicate that breeding ratio changes very little with large changes in the fraction of total power generated in the blanket. This increase in blanket power results in an increased ratio of resonance to thermal absorptions, a phenomenum which tends to offset the additional fast neutron leakage out of the blanket as blanket power increases.

An economic analysis of the effects of changing the blanket power fraction was performed to determine the optimum core-blanket power split under equilibrium operating conditions. The parameters affecting this choice are (1) fission-product poison levels in the blanket, (2) fissionproduct poison levels in the core, and (3) chemical processing costs.

Fission-product poisons in the blanket. The chemical processing of the blanket slurry accomplishes two things:

(1) The removal of bred U²³³ from the blanket system at a rate necessary to maintain the U²³³ concentration in the blanket slurry at some equilibrium value corresponding to the desired blanket power fraction.

(2) The removal of fission products from the blanket slurry.

 

If the blanket processing cycle is determined by the minimum removal rate of U²³³ for steady-state operation, a corresponding poison level in the blanket is automatically set. If the blanket chemical processing cycle is determined by the poison level and is less than the cycle determined by the above criteria, the bred fuel removed from the blanket must be fed back into both core and blanket to maintain steady-state fuel concentrations. In this analysis the blanket processing cycle in all cases was assumed to be based on the minimum removal rate to maintain steady-state U²³³ concentrations without feeding fuel into the blanket system.

Chemical processing cycle for blanket slurry. The chemical processing was assumed to be performed continuously on the reactor site. Unless otherwise specified, the fluoride volatility process is utilized as described in Article 24-3.16. The chemical processing cycle for the blanket may be calculated [3] from the equation

 



 

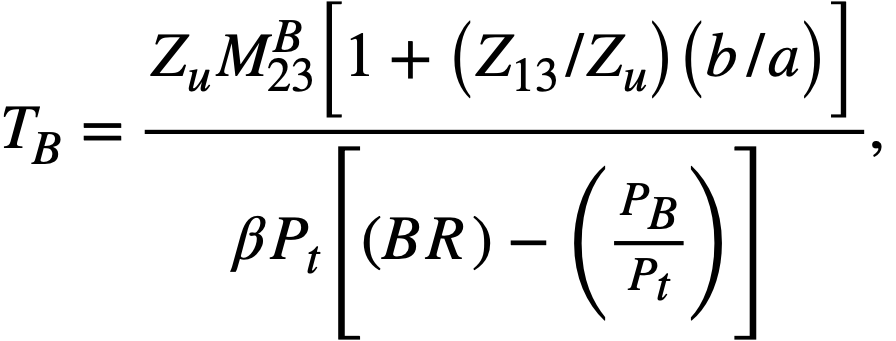
where

T_B = blanket processing cycle, days,

Z_u = removal efficiency for uranium= 0.25,

Z₁₃ = removal efficiency for protactinium= 0.04,

M₂₃^B = mass of fuel in blanket system, kg,

b/a = ratio of Pa233 to U²³³ in blanket,

β = kg of fuel burned per Mwd= 1.05(1 + α₂₃),

P_t = total power, 825 Mw,

BR= breeding ratio,

P_B = blanket power, Mw,

 

and

 



 

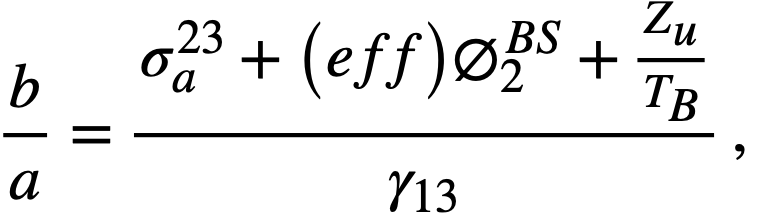
Where

 

σ_a²³(eff) = an effective absorption cross section to account for resonance and thermal absorption in U²³³,

Ф₂^BS = average thermal flux over the blanket system,

γ₁₃ = decay constant for Pa²³³.

 

The poison level in the blanket depends upon T_B, and T_B is a function of M₂₃^B, b/a, breeding ratio, and power fraction in the blanket. All these variables are interrelated. The ratio b/a is a function of T_B, but T_B is a slowly varying function of b/a due to the low value of Z₁₃/Z_u (0.16). Breeding ratio is a slowly varying function of fission-product levels in the blanket due to the heavy loading of fuel and thorium in that region. The breeding ratio is sensitive to the poison level, and thus to the chemical processing rate, in the core fuel solution. An iterative calculation procedure was required to arrive at optimum values of T_B, fission-product poison level in the blanket, and the power fraction in the blanket.

For a given chemical processing rate in the blanket, the fission-product poison level was determined from the data in KAPL 1226 [4]. Relative poisoning, RP, is defined as the absorptions in fission products per thermal fission in fuel, while the fission-product poison fraction is the absorptions in fission products per total absorption in fuel. Xenon and samarium are treated separately and are not included in the term fission products. The burnup, F, in a region is defined as the atoms of fuel fissioned per atom present in the region. The burnup Fat time Tin the blanket is calculated from

 



 

Using this relation, the relative poisoning in the blanket was determined for each processing cycle from a graph of RP versus F [4]. The RP curve used is based upon high cross sections of all fission products with the exception of a low value for Zr93.

Xenon in the blanket. Xenon is removed from the blanket by the degasser. Although the removal rate of fission-product gases cannot be determined until experimental information becomes available, a poison fraction of 0.01 was assumed for Xe¹³⁵.

Samarium in the blanket. The removal rate of samarium by chemical processing was neglected. The steady-state ratio of , using appropriate thermal absorption cross sections, is determined by the relation

 



 

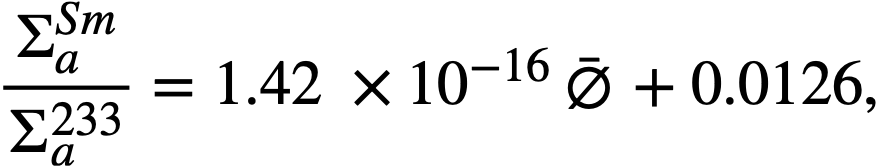
where 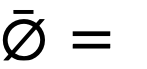 average thermal flux in the region of interest.

Fission-product poisons in the core. The level of fission products, FP, other than xenon and samarium, in the core is determined by the chemical processing cycle for the core fuel solution. The steady-state value of FP poisons in the core should be established by an economic balance between the value of improved breeding ratio and increased chemical processing costs. The relationship between the core processing cycle, T_c, and the relative poison, RP, in the core may be expressed as

 



 

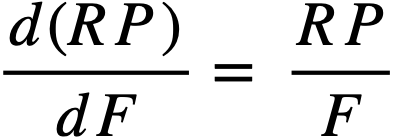
and

 



 

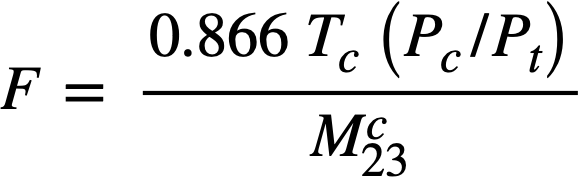
where

 

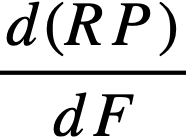 is the slope of the curve RP versus F [4],

 

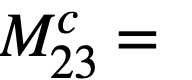total mass of U²³³ in the core system.

 

The xenon and samarium poisons in the core are determined as described for the blanket.

 



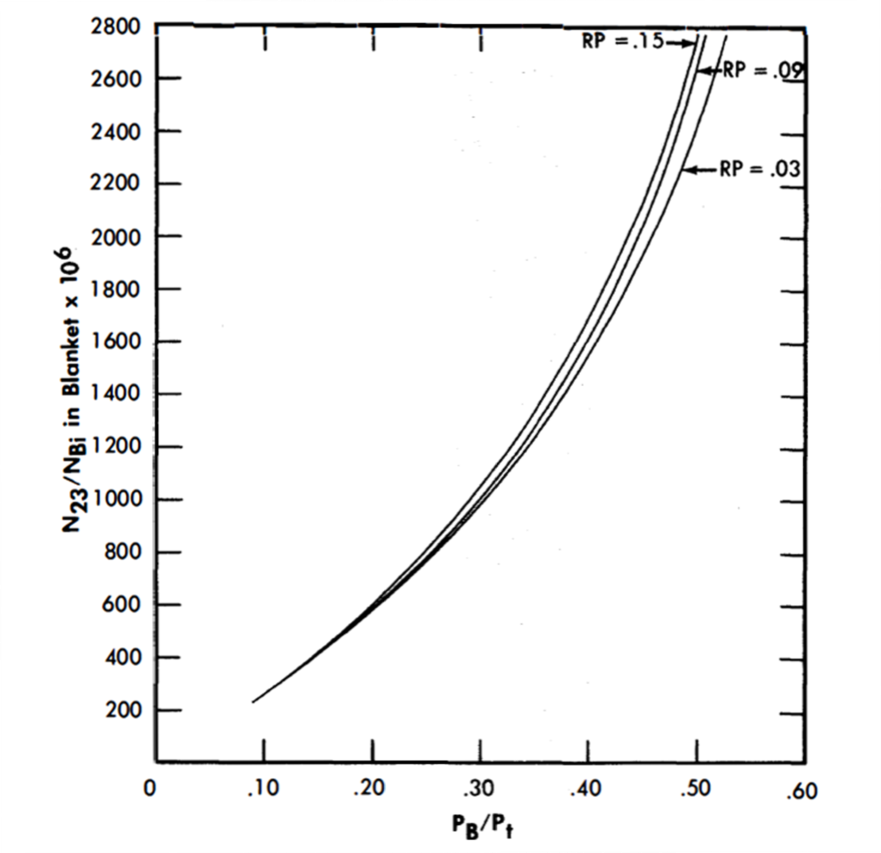
FIG. 24-5. Fuel concentration in blanket vs. P_B/P_t for two-fluid LMFR fully blanketed sphere.

 

Economic optimization. An optimization study was performed to determine the most economic power split between core and blanket systems and fission-product poison level for the core during equilibrium operation. The fuel cost items which vary with these two parameters are (1)bismuth inventory, (2)fuel inventory, (3)fuel burnup, (4)thorium amortization, (5)thorium burnup, and (6)chemical processing. Nuclear calculations specified the fuel concentrations for both core and blanket and breeding ratios. These values were then used to determine the chemical processing cycle for the blanket and the pertinent costs.

 

Nuclear calculations. The values of the parameters investigated were

 

RP (core)= 0.03, 0.09, 0.15,

P_B/P_t = 0.10-0.50.

 

Since only a relative comparison was needed, all calculations were made with a spherical core and complete 3-ft spherical blanket. The xenon poison fraction was taken as 0.01, and the samarium steady-state value was computed for each region in each case.

The fission-product poison level in the blanket cannot be determined without first knowing the blanket processing cycle. As a first approach, the breeding ratio for the hot clean conditions was used to determine the cycle time from which the RP in the blanket was calculated as described previously. The relative poison levels determined on this basis were as follows:

 

PB/Pt	RP (blanket)
10%	0.029
25%	0.048
50%	0.155

 



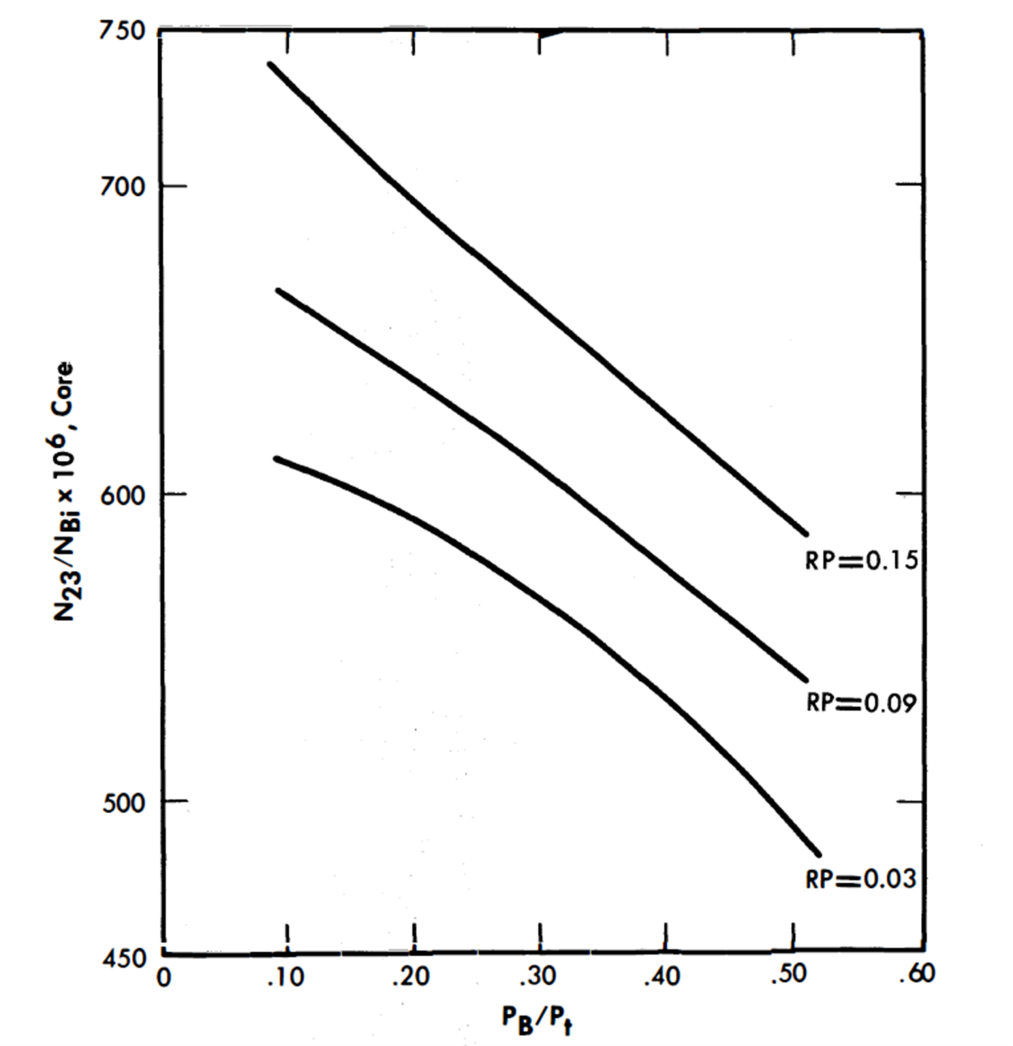
FIG. 24-6. Fuel concentration in core vs. P_B/P_t for two-fluid LMFR fully blanketed sphere.

 

All criticality calculations were performed using the specifications outlined in Article 24-2.2. Two-group diffusion theory was employed, and a two-group, multiregion code was used for solving the diffusion equations. As previously mentioned a 37-group spectral code was used to generate the two-group coefficients. The critical concentration of fuel in the core and blanket, breeding ratio, and neutron losses were determined for several power splits for each relative poison level in the core. The blanket power fraction values of 10, 33.3, and 50% were used as reference values for comparison, and the important nuclear parameters were determined from a set of parametric curves for these precise values. (Cases actually calculated corresponded very closely to the desired blanket power in most calculations.)

 

Table 24-2
Results Of Nuclear Calculations For Various Power Splits

 

Case	PB/Pt,	Relative poison in core	Relative poison in blanket	BR	1+ α23	N23/NBi x 106 (core)	M23C, kg	N23/NBi x 106 (blanket)	M23B, kg	Average thermal flux in core system	Average thermal flux in blanket system
I (a) (b) (c)	0.10	0.03 0.09 0.15	0.029	1.0256 1.007 0.978	1.132 1.132 1.132	620 664 732	368.7 395 435.4	255 255 255	53.2 53.2 53.2	5.77 x 1013 5.15 4.425	5.20 x 10l3 4.97 467
II (a) (b) (c)	0.3333	0.03 0.09 0.15	0.0475	1.007 0.993 0.978	1.132 1.132 1.132	554 599 667	215.8 233.5 260	1150 1190 1230	317 328 334	7.13 x 1013 6.40 5.71	2.61 x 1013 2.39 2.23
III (a) (b) (c)	0.50	0.03 0.09 0.15	0.155	0.980 0.959 0.945	1.135 1.135 1.135	494 542 590	154.8 170 185	2420 2670 2760	834 920 951	7.62 x 1013 6.72 6.19	1.28 x 1013 1.01 0.97

 

The nuclear parameters corresponding to these power splits are summarized in Table 24-2. Figures 24-5 and 24-6 show the variation of N₂₃/N_Bi in both the core and blanket as the blanket power fraction changes. This atom ratio of U²³³ to bismuth in the blanket ranges from 255 x 10^-6 to 2420 x 10^-6 for P_B/P_t = 0.10 to 0.50. In the core the N₂₃/N_Bi ratio decreases approximately 20% over the same range. The values of the average thermal neutron flux in the core and blanket are graphed in Figs. 24-7 and 24-8, and BR in Fig. 24-9.

 



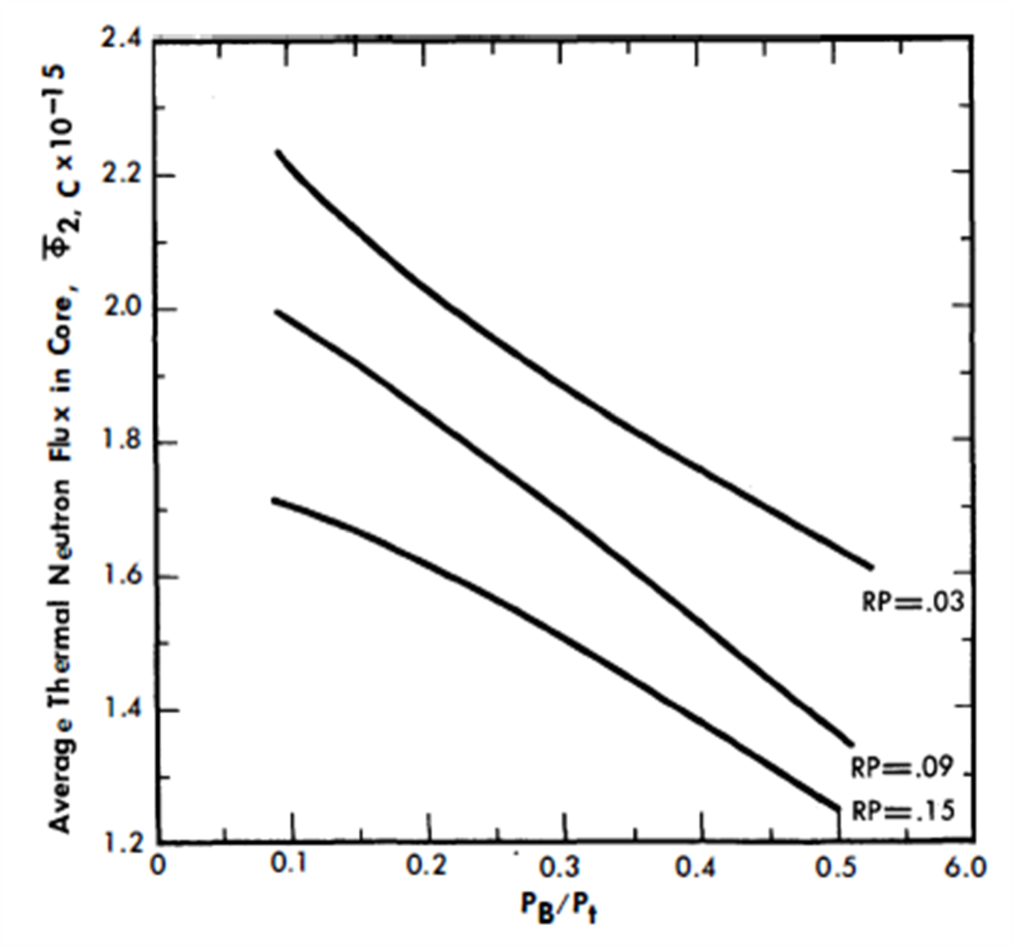
FIG. 24-7. Average thermal flux in core vs. P_B/P_t for two-fluid LMFR based on a fully blanketed sphere at 825 Mw.

 

Bismuth inventory. The primary system volumes for P_B/P_t = 0.33 and 0.50 are based on a six-loop capsule design. Each loop contains a bismuth inventory of 245 ft3 If 50% of the power is generated in the blanket, three loops contain blanket slurry and three contain U-Bi core solution. If one-third of the power originates in the blanket, two loops are devoted to the blanket system and four to the core system. If only 10% of the total power is generated in the blanket, a three-loop design is assumed for the core system, and two small loops of 125 ft3 each are used for the blanket. The reactor holdup has been estimated from the reactor drawing in Fig. 24--4. Fuel inventory volumes are summarized in Table 24-3.

Using the value of $2.25/lb of bismuth, 12% annual fixed charges, and a density of 613.5 lb/ft³ (9.83 g/cc), the annual bismuth inventory charges are

C₁($/yr) = 165.6 (V_cs + V_bs) ,

 

where

V_cs = inventory volume of core system, ft³,

V_bs = inventory volume of blanket system, ft³



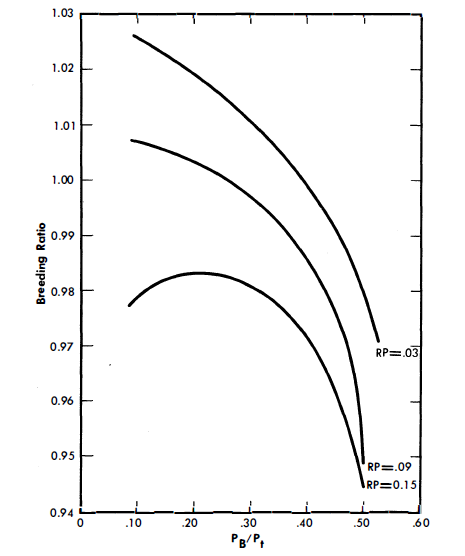
FIG. 24-8. Average thermal flux in blanket vs. P_B/P_t for two-fluid LMFR based on a fully blanketed sphere at 825 Mw.



FIG. 24-9. Breeding ratio vs. P_B/P_t for two-fluid LMFR fully blanketed sphere.

Fuel inventory. Five days' holdup of fuel from both blanket and core is assumed for the chemical processing plant. Pa233 is held up for 135 days to allow for decay to U²³³ Approximately 3% of the Pa233 remains after 135 days and is discarded with the fission-product waste. This loss, while quite small, has been included with the fuel inventory charges, which may be expressed as

 



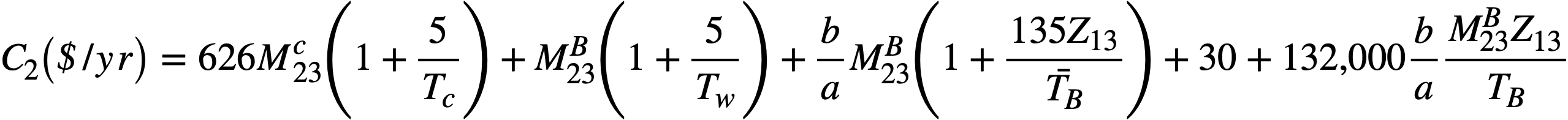
This equation assumes a 30-kg inventory of U²³³ feed material external to the reactor. The economic assumptions used in this equation are 4% fuel lease charges and a U price of $15.65/2g.

 

 

 

 

 

Table 24-3

Inventory V Olumes In Two-Fluid Lmfr

	PB/Pt= 0.10	Pt/Pc = 0.333	Pt/Pc = 0.50
Core system:
Reactor	275 ft3	275 ft3	275 ft3
External system	1640	980	735
Subtotal	1915	1255	1010
Blanket system:
Reactor	495	495	495
External system	250	490	735
Subtotal	745	985	1230
Total	2660	2240	2240

 

Fuel burnup. The annual cost of the net U²³³ fuel burned in an 825-Mw reactor, assuming an 80% plant factor, is

C₃ ($/yr) = 3.96 x 10⁶ (1 + α₂₃)(l - BR).

 

Thorium amortization charges. Assuming a cost of $42/kg for thorium and an annual amortization rate of 15% based on a 20-yr life, the annual amortization charges for the thorium are

 

C₄ ($/yr)= 6.3 M₀₂.

 

Thorium burnup. The thorium replacement costs due to burnup are calculated according to the equation

 

C₅ ($/yr) = 10,620 (1 + α₂₃) BR.



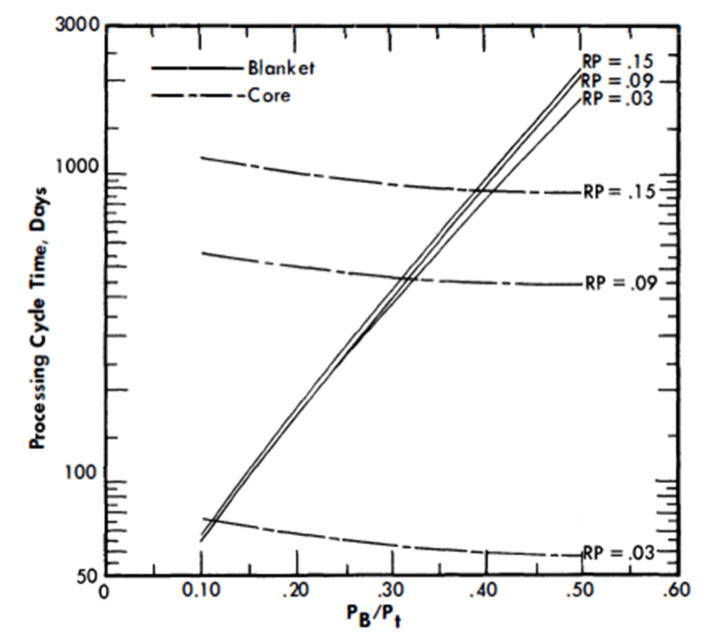
FIG. 24-10. Chemical processing cycles vs. blanket power, based on a blanketed sphere with total reactor power of 825 Mw and the removal efficiencies of Z_u = 0.25, Z₁₃ = 0.04, Z_FP^B = 0.10, Z_FP^C = 1.00.

 



FIG. 24-11. Annual fluoride volatility processing cost vs. plant throughput for 825-Mw-two-fl.uid LMFR.



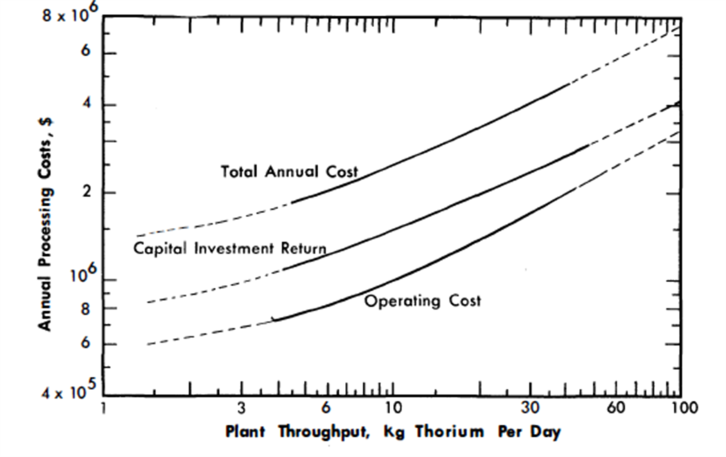
FIG. 24-12. Annual aqueous processing costs vs. plant throughput for 825-Mw-two-fluid LMFR.

 

Chemical processing costs. The chemical processing cycle time for the blanket is determined by the P_B/P_t ratio and the breeding ratio, as discussed in previous paragraphs. The processing rate for the core system is determined by the method also described previously; see Fig. 24-10. The total throughput to the fluoride volatility chemical separations plant is simply:

 



 

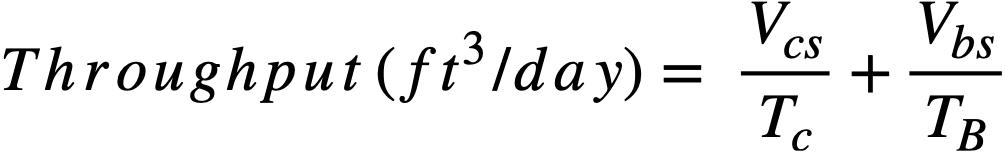
The annual processing charges based on fluoride volatility can be read directly from Fig. 24-11, a plot of annual charges versus plant throughput.

As a matter of comparison, the chemical processing charges were also computed for each case, assuming on-site aqueous processing methods. The capacity and cost of an aqueous processing plant are determined by the amount of thorium per day which must be processed. The core solution processing does not enter into the cost unless the ratio of fuel to thorium presents criticality problems in the process equipment. This situation is likely to occur for the higher power levels in the blanket. This analysis did not take this possibility into account, however, and annual aqueous processing costs were taken directly from Fig. 24-12. This design plant capacity is 35 kg/day of thorium feed.

Results of optimization. The bismuth inventory is slightly greater for the case of P_B/P_t = 0.10 than for the other two cases, because of the added primary system volume. Fuel inventory charges are not very sensitive to the relative poison level in the core, but they increase sharply with an increase in power level (Fig. 24-13). Thorium charges increase linearly with blanket system slurry volume, and fuel burnup charges increase as PB/Pt increases, as shown in Fig. 24-13.

 



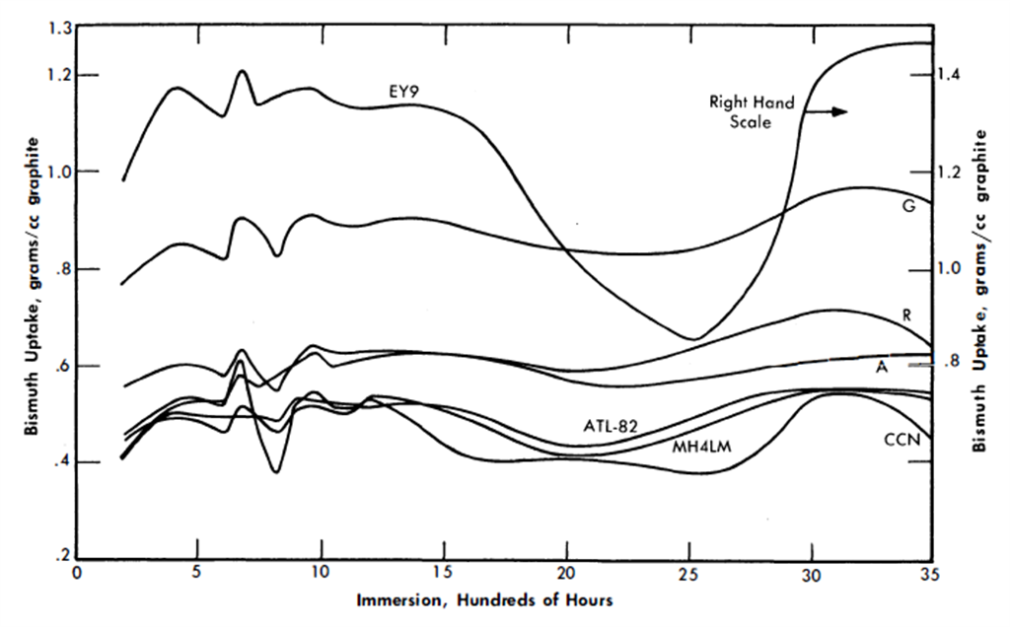
FIG. 24-13. Relative fuel costs vs. blanket power for two-fluid LMFR based on a fully blanketed sphere operating at 825-Mw with a plant factor of 80%.

 



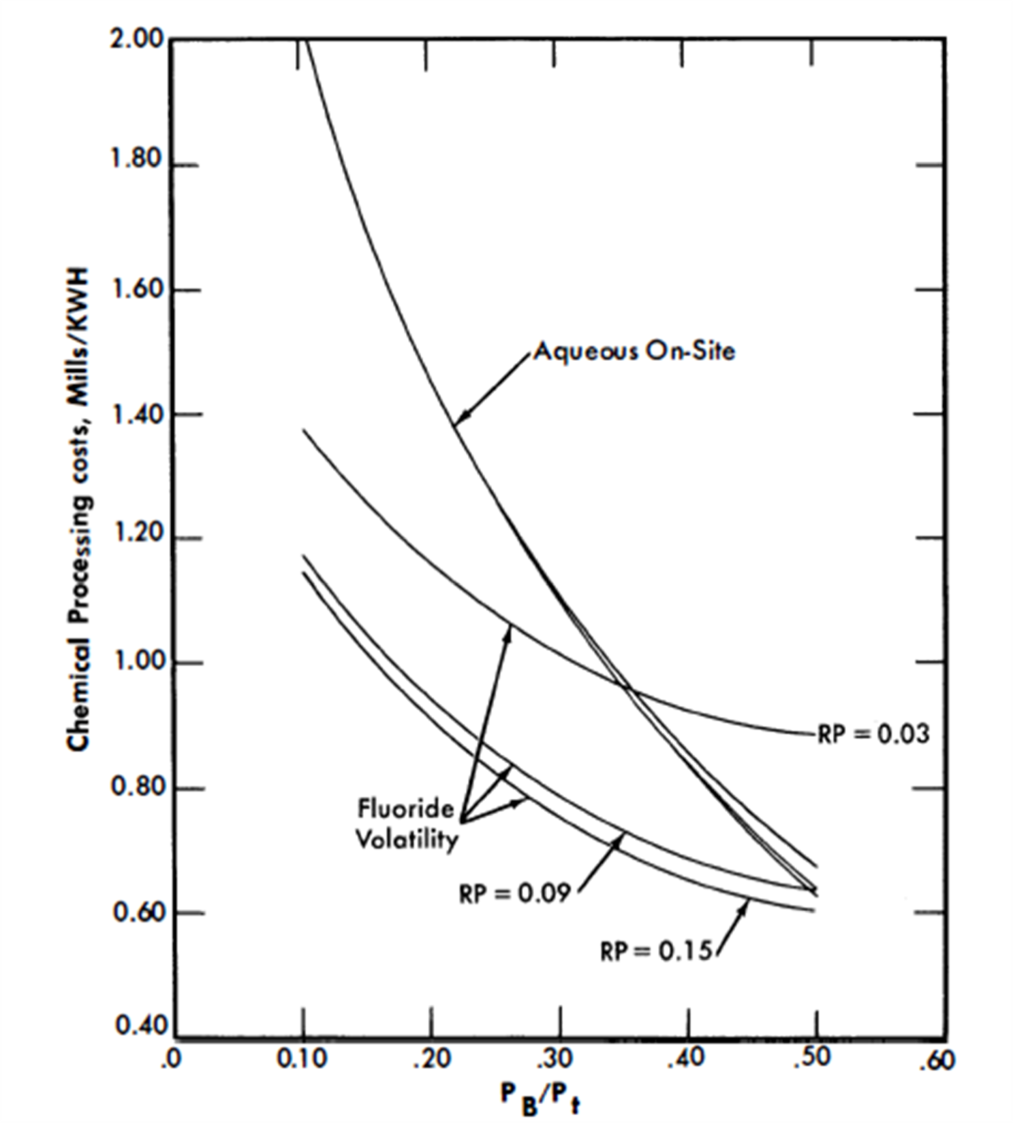
FIG. 24-14. Chemical processing costs vs. blanket power. The cycle times are based on a blanketed spherical reactor with a total heat power of 825-Mw.

 



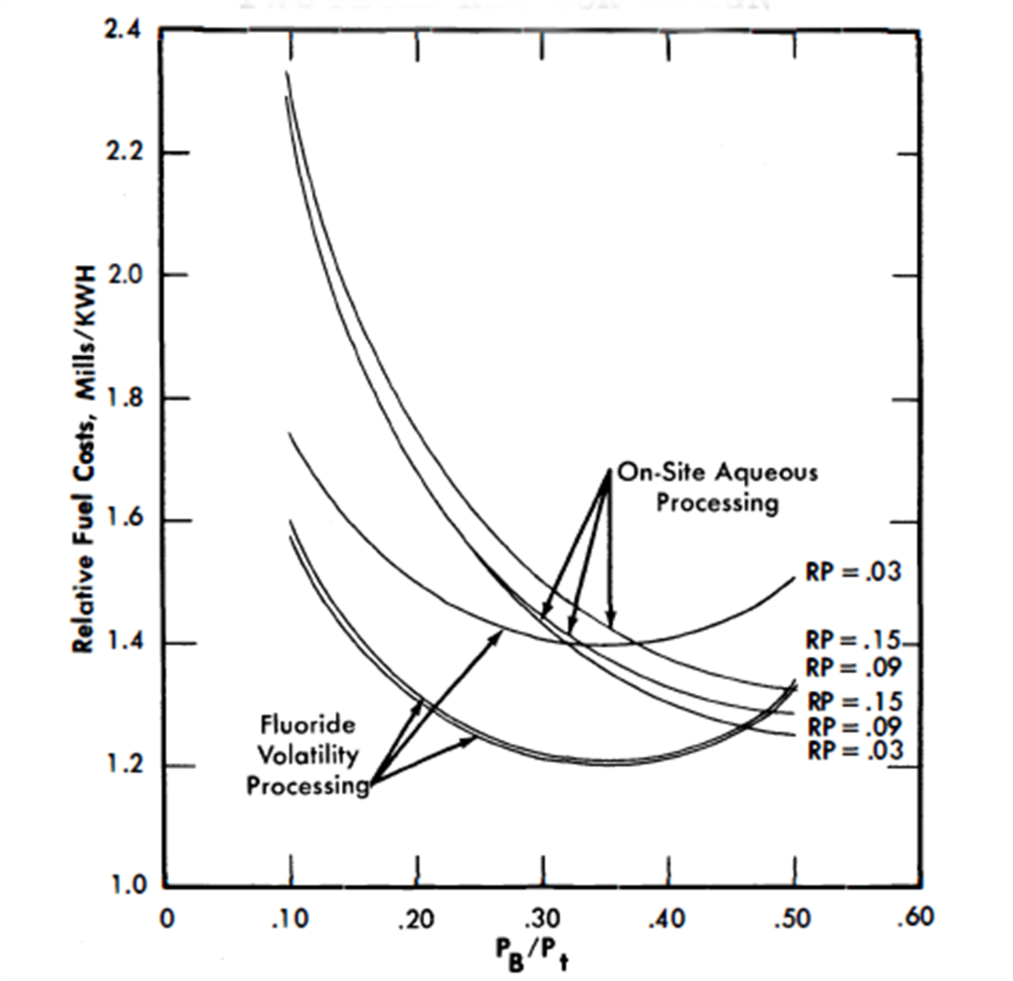
FIG. 24-15. Relative fuel costs vs. blanket power for a blanketed spherical reactor operating at a total power of 825 Mw with a plant factor of 80%.

 

Chemical processing costs drop rapidly as the power fraction in the blanket increases. The increased processing rate required to maintain a steady-state fission-product relative poison level in the core of 0.03' results in a processing cost much higher than required for RP values greater than 0.09. The aqueous processing costs appear to become essentially equal to fluoride volatility costs at a value of 50% for P_B/P_t, Further analysis would be required to determine the validity of the aqueous processing cost curve for low throughput and high N₂₃/N₀₂ ratios encountered in the cases of high blanket power. The chemical processing costs are tabulated in Table 24-4 and shown graphically in Fig. 24-14.

The results of the economic comparisons are summarized in Table 24-5 and are graphed in Fig. 24-15. (RP on the graphs refers to the relative poison level of the fission products in the core.) Figure 24-15 shows that for all values of RP a minimum fuel cost occurs for a P_B/P_t of approximately 0.33.

 

 

 

Table 24-4
Chemical Processing Costs Two-Fluid LMFR

Case	PB/Pt	Blanket process cycle, days	Slurry flow rate to chemical plant, ft3/day, Vbs/TB	RP (core)	Tc days	U-Bi flow to chemical plant, ft3/day, Vcs /Tc	Chemical plant thruput, ft3/day,	Fluoride volatility costs, $/yr x 10-6	Fluoride volatility costs, mills/ kwh	TB(Zu = 1)	Th/day to chemical plant, M02/TB	Aqueous processing cost, $/yr x 10-6	Aqueous processing cost, mills/kwh
I (a) (b) (c)	0.10	16.26	45.8	0.03	75.8	25.3	7l.1	3.03	l.37	65	325	4.20	l.90
	16.59	44.9	0.09	557	3.44	48.3	2.57	l.17	66	320	4.15	l.88
	17.08	43.6	0.15	1116	l.71	45.3	2.51	l.14	68	310	4.10	l.86
II (a) (b) (c)	0.3333	122.3	8.05	0.03	60	20.9	29.0	2.15	0.974	489	57.1	2.10	0.951
	129	7.64	0.09	446	2.81	10.5	1.65	0.747	516	54.1	2 .. 08	0.937
	136.3	7.23	0.15	900	1.39	8.62	1.58	0.716	525	53.1	2.06	0.933
III (a) (b) (c)	0.50	444.5	2.77	0.03	56.5	17.9	20.7	1.95	0.883	1778	19.6	1.38	0.625
	513	2.40	0.09	432	2.34	4.74	1.40	0.634	2052	17.0	1.32	0.598
	547	2.25	0.15	854	1.18	3.43	1.33	0.602	2188	15.9	1.30	0.589

 

 

 

 

 

 

 

Table 24-5
Relative Fuel Cost For Two-Fluid Lmfr (With Poisons-Blanketed Sphere)

 

Case	PB/Pt	Bismuth inventory, C1 x 10-3 $/yr	Fuel mven- tory, C2 x10-3 $/yr	Fuel burnup, C3 x10-3 $/yr	Thorium inventory, C4 x 10-3 $/yr	Thorium burnup, C5 x 10-3 $/yr	Fluoride volatility processing costs, Cp x10-3 $/yr	Total costs incl. fluoride vol. proc.	Aqueous proc. costs, Cp x10-3 $/yr	Total costs incl. aqueous processing
Ct, x 10-3 $/yr	Ct mills / kwh	Ct x10-3 $/yr	Ct mills / kwh
I (a) (b) (c)	0.10	440	353	-116.5	133	12.3	3031	3852	1. 75	4200	5022	2.28
	440	353	-31.4	133	12.1	2570	3477	1.58	4150	5057	2.29
	440	369	98.6	133	11.8	2510	3562	1.61	4100	5152	2.33
II (a) (b) (c)	0.3333	371	399	-31.4	176	12.1	2150	3077	1.39	2100	3027	1.37
	371	407	31.4	176	11.9	1650	2647	1.20	2070	3067	1.39
	371	429	98.6	176	11.8	1580	2666	1. 21	2060	3146	1.44
III (a) (b) (c)	0.50	371	678	89.8	220	11.8	1950	3311	1.50	1380	2741	1.25
	371	731	184.5	220	11.6	1400	2918	1. 32	1320	2838	1.29
	371	766	247	220	11.4	1330	2945	1. 33	1300	2915	1.32

 

 

24-2.5 Selection of a reference design.

The optimization study indicated that the most economic reactor design should produce one-third of the total power in the blanket system and that the relative poison in the core due to fission products should be approximately 0.09. However, several effects must be considered in relating the optimum reactor to the actual operating reactor. A geometry more realistic than the fully blanketed sphere must be considered in establishing new specifications; effects of higher uranium isotopes, Pa losses, and control rods on breeding ratio must be taken into account; and a new chemical processing cycle for the blanket, along with a new fission-product poison level in the blanket, must be calculated based upon the adjusted breeding ratio.

Geometry effects. The inability to wrap a blanket around the ends of the core requires an adjustment to the parameters for the reference design based on the calculations with a full blanket. The axial leakage out of a bare ended core and a blanket with a height 1.5 times its diameter was calculated to be 0.18 neutron per absorption in fuel. An extension of the blanket length and the addition of partial end graphite reflectors are estimated to reduce the end leakage to one-half this value. The total neutron leakage, both fast and thermal, out of the partially blanketed reactor is estimated at 0.17 neutron per absorption in fuel.

The added length of core and blanket will slightly increase the critical mass, but the required N₂₃/N_Bi ratio will decrease slightly. In order to be conservative in the fuel inventory costs, however, the critical values of N₂₃/N_Bi for the fully blanketed sphere are assumed for both core and blanket.

Breeding ratio. Higher uranium isotopes. The higher uranium isotopes, primarily U234, U²³⁵, and U²³⁶, continue to build up in both the core and blanket fuels throughout reactor life, since they cannot be separated in the . chemical plant. The relative poison due to these isotopes, however, rises rapidly at first with the buildup of U²³⁶ but increases very slowly thereafter. The return from U²³⁵ fissions almost balances for losses to U²³⁴ and U²³⁶ [4]. An average poison fraction of 0.01 for the reactor is used for the reference design.

Protactinium losses. The equilibrium Pa²³³ concentration can be computed from the relationship

 



 

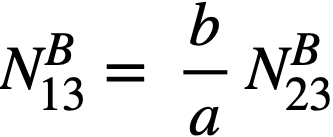
using an effective thermal absorption cross section of Pa233 based on the calculated neutron spectrum in the blanket. The relative absorptions of the Pa²³³ are very small (0.005), but they are included.

Control rods. The self-regulating properties of an LMFR have not been established at this time. An allowance of 0.01 in relative absorptions is included to account for the possibility of using a regulating rod and a small amount of shim control for normal operation. Safety rods are included in the reference design but do not affect neutron economy.

 

Reference Design Specifications

Specifications For Equilibrium Operation

Core:
Thermal power	550Mw
Electric power	210,000 kw
Diameter, inches	61
Height, inches	91.5
Fuel	U233
VBi/Vc	1.22
N23/NBi	600 x 10-6
Mass of U233 in system, kg	234
Total volume of fuel, ft3	1255
Breeding ratio, over-all	0.86
Chemical processing cycle, days	446
Volume flow rate through chemical plant, ft3/day	2.81
Mass flow rate through chemical plant, g U233/day	525
Average thermal flux in active core	1.6 x 1015
Average thermal flux in core system	6.4 x 1013
Blanket:
Thermal power	275Mw
Electric power	105,000 kw
Thickness, ft	3
Vslurry/Vc	0.5
Slurry content:
Thorium (as Th3Bi5) : 10% wt
Bismuth : 90% wt
N23/NBi (atom ratio) 1190 x 10-6
Mass of U233 in system, kg	328
Mass of thorium in system, kg	27,900
Total volume of fuel, ft3	985
Chemical processing cycle, days	200
Volume flow rate through chemical plant, ft3/day	4.91
Mass flow rate through chemical plant, kg of Th/day	140

 

Fission-product poisons. The adjustment of breeding ratio to correspond to the effects outlined above changes the required chemical processing cycle for the blanket system. This change in T_B also changes the equilibrium value of fission products in the blanket. Proper adjustments result in a blanket processing cycles of 200 days (assuming Z.,= 0.25) and a fission product poison fraction in the blanket of 0.039 (RP in blanket= 0.15).

 

Neutron balance. The neutron losses proportional to one absorption in U²³³ are listed below:

 

Absorptions in: U233	1.000
Th	0.860
C	0.025
Bi	0.050
Xe135	0.010
Sm149	0.017
Fission products	0.073
Higher isotopes	0.010
Control rod	0.010
Pa233	0.005
Leakage	0.170
Total	2.230

 

 

24-3. Systems Design

190/ 24-3.1 General.

Systems design covers all of the reactor plant external to the reactor, except for chemical processing. The reactor plant includes the steam generator, but not the steam system or its auxiliaries. The principal purpose of the systems is to transport heat from the reactor and generate steam. They also provide supporting functions, such as shield cooling, uranium addition, etc.

The primary system consists of six heat transport loops, each consisting of a pump, a heat exchanger, check valve, and interconnecting piping. The hot-leg temperature is 1050°F; the cold-leg temperature 750°F. In each of the intermediate heat exchangers, heat is transferred from the bismuth to the intermediate fluid, sodium. There are six intermediate heat transport loops, each containing a pump, steam generator, and interconnecting piping. The hot-leg temperature is 1010°F; the cold-leg temperature 680°F. Steam is produced at 2100 psia, 1000°F.

Selection of the above parameters was a problem involving consideration of the steam plant as well as the reactor plant. The primary system temperatures were first fixed by using the largest ∆T considered likely to prove practical.

The temperature approach of the intermediate heat exchanger was set at 40°F, resulting in a sodium hot-leg temperature of 1010°F. To provide the close approach necessary for steam temperature stability, the steam temperature was set at 1000°F. A steam pressure of 2100 psig was picked to correspond with 1000°F.

Shifting the sodium cold-leg temperature redistributes heat-transfer surface between the intermediate heat exchanger and the steam generator. However, it seems desirable to favor making the intermediate heat exchanger small to cut down on fuel inventory. For this reason, the sodium cold-leg temperature was established at 680°F.

24-3.2 Plant arrangement.

Plant arrangement starts with positioning the primary system relative to the reactor, and this is determined by seven principal considerations: (1) reactor design, (2) plant operation, (3) maintenance, (4) operational limitations of major components, (5) structural integrity of piping, (6) economics, and (7) safety.

A preliminary analysis of the two reactor concepts, single-fluid and two-fluid, resulted in the decision to use three external loops for the single-fluid and six for the two-fluid reactor. For both these alternates the maintenance philosophy selected was that of removal and replacement by horizontal transfer of a complete primary loop upon failure of any major component in the loop [5]. Thus, for arrangement purposes, the primary loops assume the shape of a rail-mounted horizontal containment vessel, or capsule, sized to contain all loop components. The height of the capsules relative to the reactor is dictated by an economic balance between height or elevation costs and pump net positive suction head.

The arrangement for the two-fluid reactor with six primary loops is shown in Figs. 24-16 and 24-17.

In plan, the primary loops were located radially around the reactor, Fig. 24-16. A minimum length of interconnecting pipe between the reactor and the loops was used because of high fuel inventory costs. This latter consideration ruled out shielding of any appreciable thickness between the reactor and the loops. Maintenance access doors and other shielding around the outside of the loops was sized for source conditions 6 to 8 hr after shutdown of the reactor to permit access by maintenance personnel at that time into the annular area.

With the primary loop arrangement established, the next problem was location of the intermediate system. Since this system is the connecting link between the primary systems and the steam turbines, it must be located between them. The turbine is above ground level for gravity drainage of condenser cooling water, and the primary loops are below ground level for economy of shield costs. The path taken by the intermediate system can be either a high-level path, immediately up from the primary system, or a low-level path, immediately down from the primary system, and then horizontally to an area outside the primary system area.

The intermediate system in this arrangement follows the high-level route to the steam plant. Sodium lines are brought straight up to an annular area around the reactor maintenance chamber. Since access to this chamber will not be permitted during reactor operation, a heavy shield wall is not required around the chamber.

 



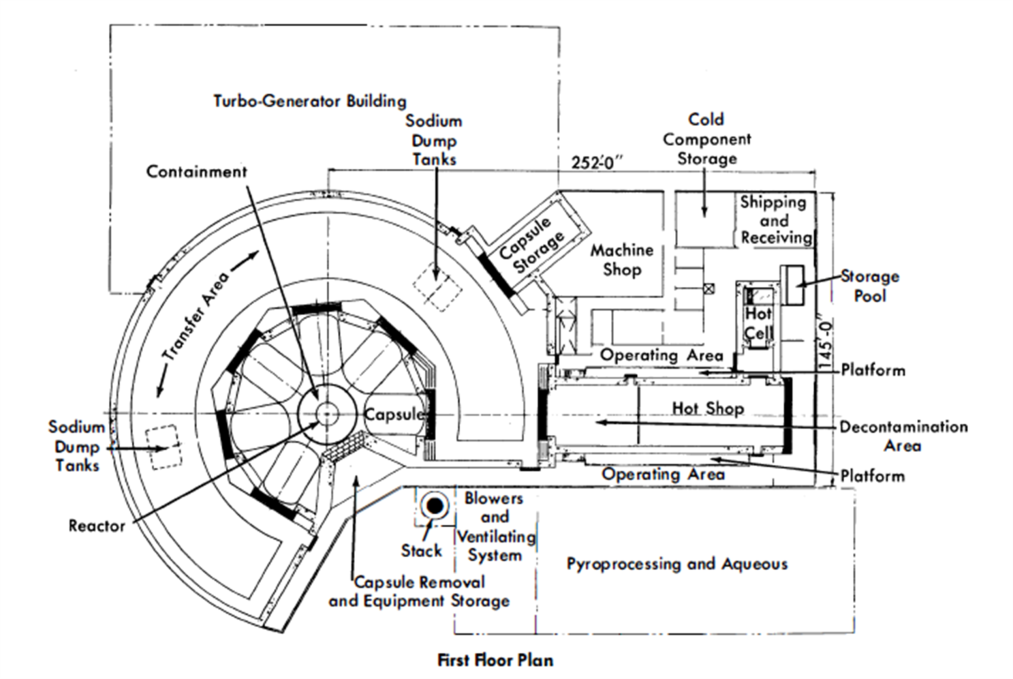
FIG. 24-16. LMFR-6: Capsulate loop conceptual plant layout.

 

Within this annulus are the sodium pumps and the steam generators. Final layout of this equipment will require considerable ingenuity, but it is feasible. Steam lines will cross the roof of the reactor building to the turbine building.

Because the primary loop hot maintenance shop for this concept serves such specialized functions, its usefulness for maintenance of chemical processing equipment is doubtful. Accordingly, the chemical processing facilities for this two-fluid six-loop plant, together with its supporting hot and conventional laboratories, fuel addition and other systems, are located in a separate building.

The turbine building is of conventional construction and will be in all essential respects identical for both plants.

Startup heating switch gear, gas heating and cooling systems for the reactor and dump tanks, inert gas storage systems, control rooms, and other auxiliaries are located relative to the above systems as logically as possible in the light of their functional requirements.

 



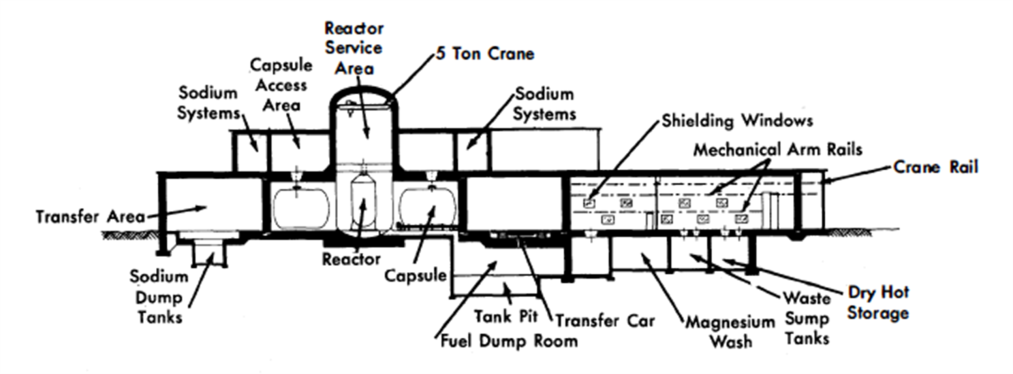
FIG. 24-17. LMFR-6: Capsulate loop conceptual plant elevation.

 

With respect to contamination control the basic philosophy is (1) controlled access to areas having different order of magnitude activity levels and (2) controlled circulation of ventilating air to assure flow from low- to high-level activity areas. For guidance in achieving these objectives a rough scale of activity levels has been proposed, as follows:

Class # 1 - conventional steam turbine plant, personnel monitored.

Class # 2 - uncontaminated areas of nuclear plant, personnel monitored.

Class # 3 - potentially contaminated areas, personnel closely monitored; e.g., shield cooling, reactor and dump tank heating and cooling, hot shop operating area.

Class #4 - low activity, accessible by closely monitored personnel only under favorable conditions; e.g., exhaust blower room, hot chemical laboratory.

Class #5 - medium to high activity, accessible by closely monitored personnel only after executing standard decontamination procedures; e.g., hot maintenance shop.

Class #6 - high activity, no access during life of plant except after extended shutdown and special decontamination; e.g., chemical processing and chemical hot cell.

Class # 7 - very high activity, no access by personnel during or after life of the plant; e.g., primary loop and reactor areas.

24-3.3 Primary system.

The LMFR primary system is designed to remove up to 825 Mw of heat from the reactor. The primary system consists of six separate heat transport loops.

The fuel stream enters the bottom portion of the reactor vessel at a minimum bulk temperature of 750°F, and flows upward through the core, where fissions within the fuel cause the fluid to undergo a temperature rise of 300°F, resulting in a maximum fuel temperature of 1050°F. Upon leaving the core, the fluid passes upward to a degassing area, where volatile fission products are removed from the fuel stream. The reactor discharge consists of a header which splits the fuel flow into the primary heat­transport loops.

The primary loop piping, 20 in. in diameter, is sized to obtain a maximum fuel velocity of 10 fps.

From the degassing area discharge, each fuel stream flows to the suction of a variable speed centrifugal sump type pump. Each pump is designed to deliver about 9000 gpm at 20-ft head of pumped fluid. To obtain a reasonable pump speed, the net positive suction head requirement is

11.5 ft. A gas pressure (helium) is maintained over the pump sump to prevent flooding of upper parts (motor windings, cooling system, etc.) of the pump.

From pump discharge the fuel stream flows to the tube side of a U-tube U-shell intermediate heat exchanger in which the fuel stream gives up heat to the intermediate fluid, sodium. Upon discharge from the intermediate heat exchanger, the fuel solution flows into the reactor.

To meet safety requirements, the reactor and the major components of the primary loops are enclosed within containment vessels. The containment vessel which houses the pump and heat exchanger of each primary loop is a cylindrical capsule, 20 ft in diameter by 30 ft long, including 2:1 elliptical heads. The capsule is equipped with access holes such that certain maintenance operations may be performed [5]. The reactor containment vessel is a right circular cylinder 30 ft in diameter, with a hemispherical top head and a flat bottom head. Access holes are provided in the vessel for maintenance operations.

Each heat-transport loop is provided with four dump tanks which receive the loop and a portion of the reactor volumes. The tanks are sized and arranged to prevent a fast chain reaction. The primary loops are filled from the dump tanks by means of small electromagnetic pumps. These pumps also promise a means for agitation of the fuel.

Two dump lines, each with a maintainable valve, connect each loop with the dump tanks.

The bismuth charge system consists of a bismuth melt tank, filter, valves, and piping to the dump tanks.

The proposed material of construction exposed to primary fluid is 2¼% Cr-1% Mo steel.

24-3.4 Intermediate system.

The intermediate system, which also consists of six separate heat-transfer loops, utilizes sodium as the heat-transfer medium. AH material of construction of the intermediate system, except the steam generator, is 2¼% Cr-1% Mo steel. The steam generator, which is designed for high-pressure, high-temperature service, is constructed of type-304 stainless steel. The intermediate piping (12-in. schedule-30) is sized for a maximum sodium velocity of 17 fps.

Sodium flowing at 11,000 gpm enters the shell side of the intermediate heat exchanger (which is a U-tube, U-shell unit containing 2400 5/8-in.-OD tubes with an average length of 21 ft) at 680°F, flows countercurrent to the fuel stream, and exits from the heat exchanger. Sodium flows to the suction of a variable speed centrifugal sump type pump. Each intermediate pump is designed to deliver 11,000 gpm at 180-ft head.

From pump discharge sodium flows to the shell side of the steam generator. The steam generator is a U-tube, U-shell “once-through” type unit which is constructed of type-304 stainless steel. The unit consists of 530 1/2-in.-OD tubes with an average length of 65 ft. The shell OD is 29 in. and the over-all length is 68 ft.

Sodium flows countercurrent to superheated steam, boiling water, and feed-water in the steam generator and gives up heat which produces 1,100,000 lb/hr of superheated steam at 2250 psig and 1000°F.

From the steam generator sodium flows to the intermediate heat exchanger inlet to complete the cycle.

In addition to the components listed above, auxiliary components are necessary to obtain proper function of the intermediate system. An expansion tank is located at the highest point of each intermediate loop. This tank serves as a cushion for pressure surges, a surge vessel for thermal expansion of sodium, and suction head for the pumps. The lowest point of each intermediate loop is connected by pipe and dump valves to a sodium dump tank which receives the inventory of the respective loop. Sodium is replaced in the loop by a small electromagnetic pump which takes suction from the bottom of the dump tank. A plugging indicator and a cold trap are provided to determine sodium oxide concentration and to maintain the oxide concentration at low levels.

In the event fission-product “hangup” occurs in the intermediate heat exchanger, fission product activity will generate heat within the metal. To prevent excessive metal temperatures, cooling must be provided when the unit is drained. This cooling is accomplished by providing removable sections of insulation which, when removed, will permit heat to be dissipated by radiation, conduction, and convection heat transfer. Flow control of the intermediate system will be by the variable speed pump drives. This method of control should provide a reasonably constant steam temperature and pressure.

24-3.5 Reactor heating and cooling system.

The reactor must be preheated prior to operation and for outgassing purposes. The required temperature for outgassing the graphite is 1000°F. To achieve preheating, hot helium gas will be circulated through the close-fitting jacket or double containment which creates an annulus surrounding the reactor vessel. During the preheating phase, helium gas will be pumped from one of two blowers, pass through an electric resistance heater, be introduced at the bottom of the annulus, pass up around the reactor vessel giving up its transported heat to the cooler surface, and return by ducting from the upper end of the containment to the blower suction.

When, for any reason, it becomes desirable to shut down the reactor and dump the primary system, reactor cooling must be provided to remove decay heat generated by fission-product hangup within the reactor after dump. This is necessary to prevent internal temperatures from exceeding design limits. The system as just described provides cooling by opening a valve to bring a finned tube helium-to-water heat exchanger into the cycle and by closing a stop valve to remove the gas heater from the gas flow path.

Helium system design pressure and temperature will be 5 psig and 1050°F. The entire loop is of all-welded construction to minimize helium leakage and leakage of volatile fission products should a rupture of the reactor vessel or piping give volatile fission products access to this loop.

24-3.6 Dump tank heating and cooling.

When fuel is drained from the primary system into the dump tanks, fission-product decay produces heat within the fuel which must be removed to prevent dump tank metal temperatures from exceeding design limits.

Cooling is accomplished by circulating helium at 140 psig through a narrow annulus around each dump tank. Helium which has removed heat from the dump tanks passes through a finned-tube heat exchanger and gives up heat to river water. Circulation of helium is provided by six 14,000 cfm blowers, each rated to provide a head of 18-in. water. Three standby blowers are also provided. Helium piping is arranged such that four dump tanks are serviced by one blower.

To preheat the dump tanks and to maintain their temperature at a level such that fuel precipitation does not occur, electric heaters are paralleled with the heat exchanger such that the same piping system serves for heating or cooling. The heaters or heat exchangers may be brought into or taken off the cycle by valving.

24-3.7 Startup heating system.

Prior to power operation, the LMFR heat-transport system must be preheated to about 800°F. The reactor and the primary dump tanks are preheated by electric furnaces and circulating helium. The remainder of the heat-transport systems, i.e., primary pipe, intermediate heat exchanger, intermediate piping, dump tanks, expansion tanks, steam generator, and the steam system pipe and components, are preheated by induction heaters.

Since 2¼ Croloy and stainless steel are nonmagnetic, a thin sheet of carbon steel will be required under areas where induction heaters are applied.

24-3.8 Primary inert gas system.

Inert gas is used in the LMFR primary system to cover all free liquid metal surfaces and to provide a gas seal within the pumps.

Helium, by virtue of its very low activation cross section and inertness, is utilized as the cover and seal gas for the primary system. It is stored at 200 psig in a storage tank and is piped via pressure-reducing valves to the pump, dump tanks, and reactor. Since relatively small quantities of helium will be used, it is expected that waste helium will be discharged via the off-gas system to the stack.

Since commercial helium is sufficiently pure for use in an LMFR, no purification will be required.

24-3.9 Intermediate inert gas system.

Nitrogen is used in the LMFR intermediate system to cover all free sodium surfaces and to provide a gas seal in the pump. It is stored at 200 psig in a storage tank and is piped via pressure-reducing valves to the pumps, expansion tanks, and the dump tanks. Used nitrogen is discharged to the stack.

Commercial nitrogen must be purified prior to use in the intermediate system. Purification is accomplished by bubbling nitrogen through several tanks containing NaK.

24-3.10 Shield cooling.

The concrete surrounding the primary cells serves as a shield from the neutrons and gammas leaving the primary fluid. In the absorption of these neutrons and gammas, considerable heat is generated within the concrete. To hold temperatures and thermal gradients within the concrete to reasonable limits, a cooling system must be utilized. This cooling system consists of panel coils embedded about 6 in. within the concrete shield. High-purity water, flowing inside the panel coils, removes heat from the concrete and prevents temperature damage to the concrete. The closed, high-purity loop which rejects heat to river water is designed for a maximum heat load of 6 Mw. One pump of 900-gpm capacity provides circulation for the closed water loop. Flow control valves proportion the flow to the various panels such that panel coil outlet temperatures are equal.

A dump tank for the closed loop (about 300 ft³) is located beneath the panel coils, so that the coils may be gravity drained. Water is returned to the closed loop by means of gas pressure. In the event it is necessary to dispose of the water in the closed loop, it may be drained from the dump tank to the radioactive waste disposal system.

24-3.11 Reactor cell cooling.

Instruments located within the reactor containment vessel must be kept at a relatively low ambient temperature. To maintain the ambient temperature, a “fin fan” cooling unit is attached to the containment vessel. Helium, which fills the containment vessel, is circulated by a blower located within the cooling unit. The circulating helium removes heat from the containment vessel and transports it to the finned coil, where it is transferred to water which is taken from and returned to the closed shield cooling circuit.

24-3.12 Capsule and reactor room cooling.

The containment capsules and the reactor are located in a large room. The ventilation requirements of this area are dependent upon heat losses from the primary loop containment capsules.

Ventilation is provided by locating air intake louvers at several points around the room. An air fan provides circulation of air around the capsules and removes heat, which is discharged to the stack. A radiation monitor continuously monitors the air discharge. In the event radiation tolerance levels are exceeded by the air discharge, the cooling air will be re-circulated to the reactor and capsule room until the source of radiation is determined.

24-3.13 Raw water system.

The raw water system is the final waste heat sink for the entire plant. River water, which is screened and treated, is piped beneath the turbine-generator building. The systems which require river water, i.e., turbine condenser, shield cooling, reactor cooling, dump tank and pump cooling, take suction from this pipe and discharge to a similar one which returns the heated water to the river. Where possible, river water flows tube side in heat exchangers, to facilitate cleaning.

24-3.14 Instrumentation and control.

The purpose of the control system in this plant is to provide safe and stable operation while following the loads imposed by the utility system. The plant follows the turbo generator. Loads on the turbo generator are set by the utility.

A load change will appear in the steam system as a change in throttle valve position and, therefore, a change in steam flow and pressure. The feed-water controllers at the inlet to the steam generators will sense these changes and operate to maintain steam pressure constant. The steam flow could also provide an anticipatory signal to the primary and intermediate system pumps to change their speed to suit the load.

The reactor will have a negative temperature coefficient of reactivity. Thus, it will try to maintain its average temperature constant during load changes. The temperature will change from time to time as reactivity changes. To take advantage of the negative temperature coefficient, the average temperature of the reactor will be set at a constant value.

Programming of flow rate in the primary and intermediate loop is uncertain. Cost estimates for pumps and control equipment were based on the premise that speed of the pumps would be varied. This might be necessary to avoid thermal stresses during transients.

 



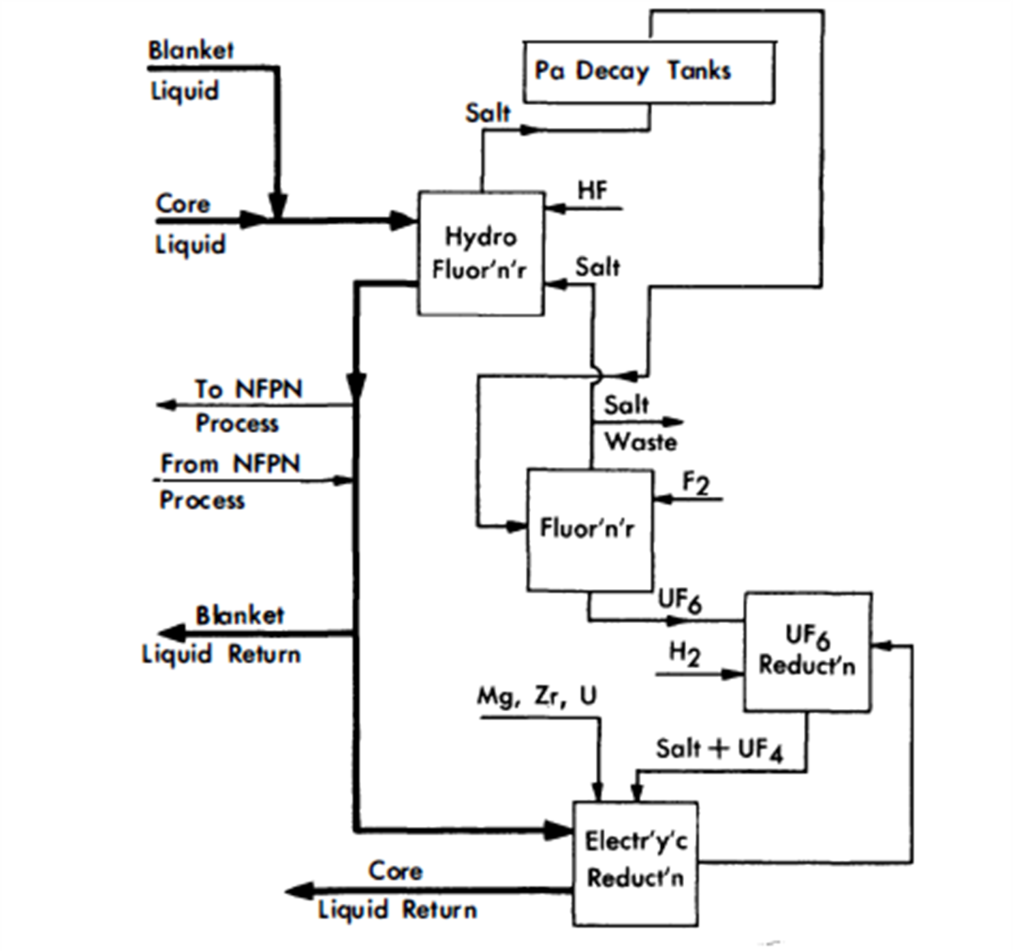
FIG. 24-18. Fluoride volatility processing of core and blanket.

 

24-3.15 Maintenance.

The maintenance of the reactor and primary system components will be completely remote, because of the high levels of radioactivity of the circulating fuel. The entire plant and reactor system are arranged for remote maintenance [5].

24-3.16 Chemical processing.

The pyro process chosen for this economic study is the fluoride volatility method applied to a two-region reactor. Work of adapting this process to bismuth fuel processing is presently under way at Argonne National Laboratory. Figure 24-18 presents the main steps in this process. As shown, the process may be used for either blanket or core liquid. When the plant is processing core liquid the basic steps in this process are (1) hydrofluorination to oxidize uranium and some fission products, (2) transfer of the oxidized material to a fused salt phase, (3) fluorination of the salt carrying the uranium and fission products for separation of uranium as volatile UF₆, (4) reduction of the UF₆ to UF₄ by H₂ in a fused salt phase, and (5) reduction of UF₄ to uranium metal and transfer into the metal phase (bismuth).

The volatility method can be conveniently used to process a thorium bismuthide blanket. The process must be preceded by a phase separation step which separates the thorium bismuthide solids from the liquid carrier bismuth (Fig. 24-19). The modification of the core liquid process flowsheet is as follows: (1) salt effluent from the hydrofluorination step must be stored in order to achieve Pa decay to uranium, and (2) the bismuth liquid is returned to the blanket head end process without the addition of uranium.

 



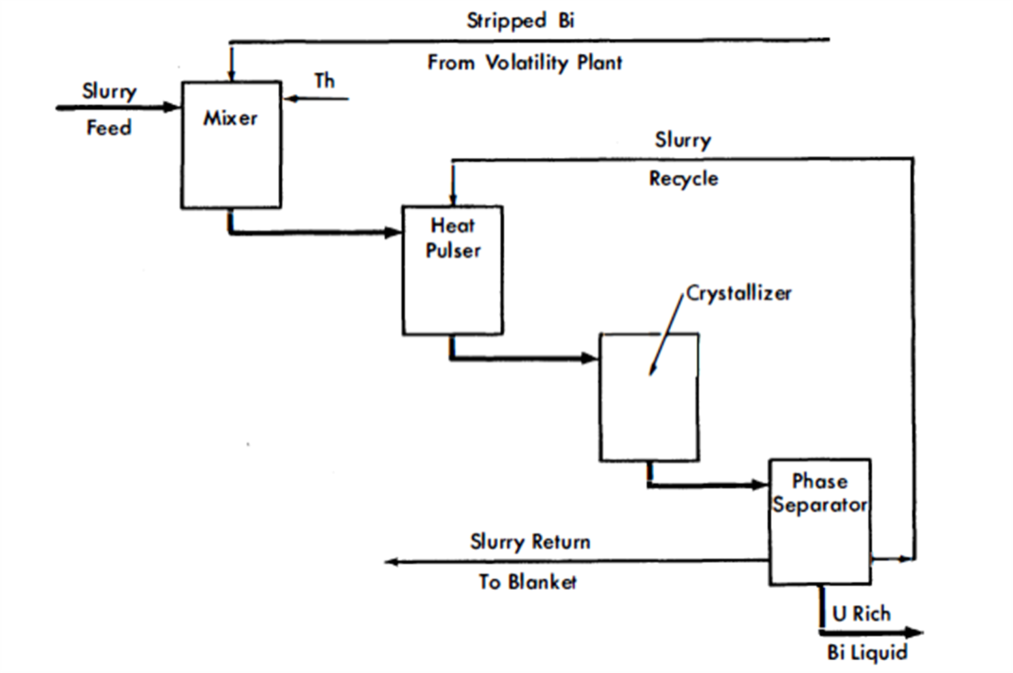
Fm. 24-19. Head end processing, bismuthide slurry.

 

 

Certain of the fission products are not removed by volatility processing. These may be removed by zinc precipitation (Fig. 24-20). This process requires that the bismuth feed be free of uranium, and the volatility plant provides such a bismuth feed stream.

The head end process transfers bred uranium, protactinium, and fission products out of the solid phase portion of the slurry and into the liquid phase. After this step the two phases are partially separated. A liquid portion transferred to the volatility plant carries bred uranium, protactinium, and fission products with it for stripping with HF. The stripped liquid bismuth is returned to the head end plant for mixing with fresh slurry feed. The head end process is not 100% efficient; i.e., the uranium and protactinium are not completely removed from the slurry before reconstitution and return to the blanket region. This problem has been examined in some detail and was taken into account in determining economics.

24-3.17 Turbine generator plant.

A flow of 3,330,000 lb/hr of superheated steam at 2100 psi and 1000°F is delivered to the turbine. The generator has a gross output of 333,000 electrical kw, and the condenser removes 1.677 x 10⁹ Btu/hr at 1.5 in. of mercury absolute, thus giving a gross heat rate of 8450 Btu/kwh. About 18,000 kw of electrical power is used for the various pumps and auxiliary systems in the plant, making the net output 315,000 kw. Therefore, the net heat rate is 8940 Btu/kwh, which corresponds to an efficiency of 38.2%.

 



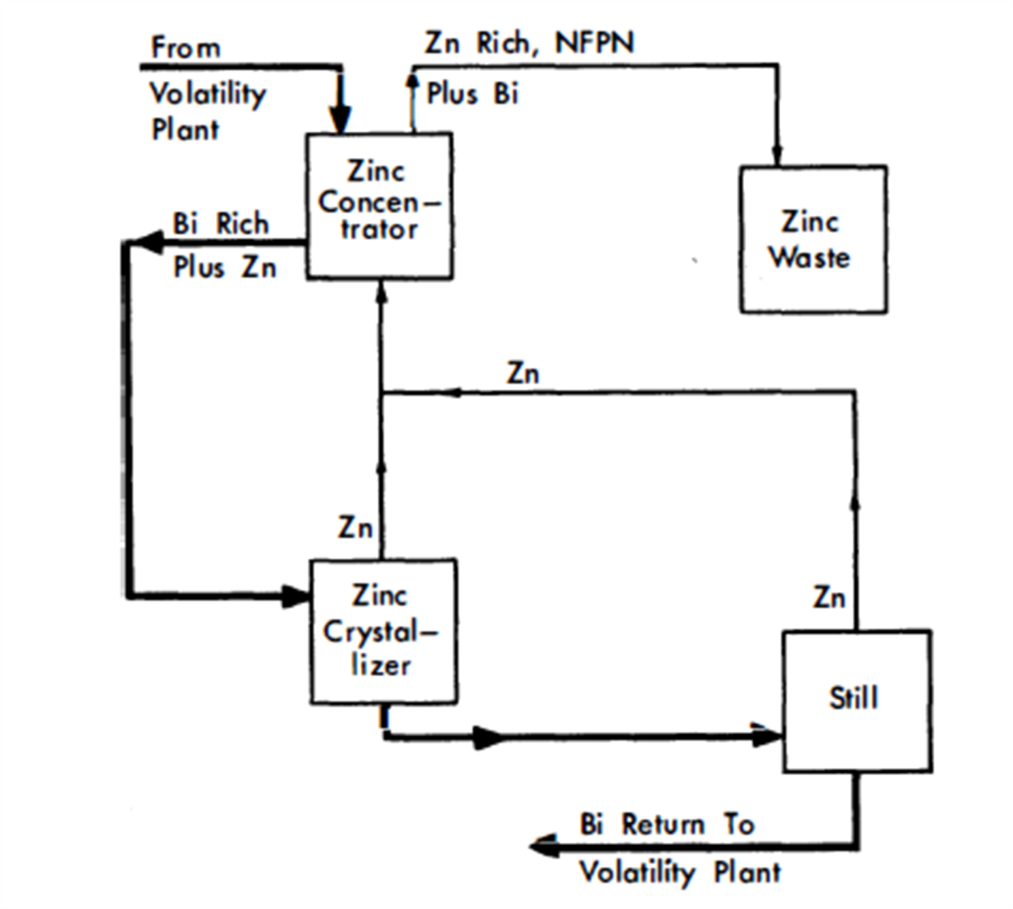
FIG. 24-20. NFPN fission-product removal.

 

At full load there are 1,825,500 lb/hr of steam leaving the turbine and being condensed in the condenser. Also, 113,800 lb/hr of water from various parts of the cycle are being cooled by the condenser. The total head load on the condenser is 1.677 X 109 Btu/hr. The condenser cooling water enters one water box at 70°F and leaves the other at 80°F.

24-3.18 Off-gas system.

The actual design and efficiency of any conceptual degasser are as yet unknown quantities, and a knowledge of these important details will have to wait until in-pile loops have provided sufficient data.

The off-gas system will consist of a cooler followed by a series of storage bottles. Gaseous fission products that have been separated from the liquid bismuth in the degasser are first sent through a cooler which offers a residence time of about a day, or enough for most of the short-lived isotopes to decay. From the cooler, the gasses are compressed into storage bottles, each capable of holding 30 days' accumulation. The storage bottles will each be 4.25 ft³ in volume, and at 212°F and 60 psia at the time of disconnection from the compressor.

Some sweep gas may be included in the above gas stream, but the present design philosophy indicates that no extra sweep gas should be required; however, if some sweep gas is required for efficient degasser operation, this gas could be obtained by a recycle of previously removed gas. This recycle sweep stream would most probably be taken from the storage bottles after sufficient cooling.

The gas in the storage bottles may be vented to the atmosphere after 90 days of storage, since then only the K:r8 5 activity is still present in appreciable amounts, and this can be released provided there is sufficient dilution. However, the most probable course of action will be to process the stored off-gas through a gas separation system, where the valuable K:r8 5 will be recovered.

24-4. Single-Fluid Reactor Design

24-4.1 General description.

The single-fluid LMFR concept has been investigated to determine the characteristics and economic attractiveness of this design. In general, the core consists of a large array of solid moderator blocks stacked to provide the desirable geometry of a cylinder. Vertical cylindrical channels are drilled through the moderator to allow circulation of the liquid metal slurry containing both the fuel and fertile material. The fission heat generated in the fuel-coolant stream is transported by forced convection to heat exchangers external to the reactor vessel. The unique feature of this concept is that only one coolant, the slurry, is used for removing heat from all parts of the reactor. The desired slurry-to-moderator ratio is achieved by selecting the appropriate combination of channel size and spacing.

24-4.2 General specifications.

The general specifications for the system affecting reactor design are tabulated below:

Power	825 Mw (thermal)
Slurry temperature:	315 Mw (electrical)
Tin	750°F
Tout	1050°F
Maximum slurry velocity	10 fps
Fuel	U235 or U233
Fertile material	Thorium
Moderator material	Graphite or BeO
Slurry carrier	Bismuth or lead
Slurry-to-moderator ratio	Variable
Fertile material content in slurry	Variable
Core geometry	Cylinder
Core size	Variable

24-4.3 Parametric study.

A parametric study was performed to determine the optimum nuclear parameters for a single-fluid concept. The variable parameters investigated and their range of values are:

 

Slurry-to-graphite ratio, V_s,/V_c= 0.05 to 1.0,

Fertile material content, g/kg of Bi= 0 to 80,

Equivalent bare reactor diameter, D, ft= 10 to 20.

 

The choice of fuel for the first full-scale LMFR will depend upon the availability of U²³³, which is much more attractive than U23 5 because of better neutron economy, and a sufficient quantity for fueling an LMFR may be available in 10 to 15 yr. In the early stages of this study, however, U²³⁵ was arbitrarily chosen as the fuel for the parametric study. The selection of the reference design should be valid for either fuel.

In each case the critical concentration and conversion ratio were determined by multigroup diffusion theory, using 37 neutron energy groups. To handle the large number of calculations, a digital computer was used once the range of values for the parameters was established by a series of criticality calculations by hand.

The use of BeO as a moderator has the advantage of reducing the core size because of improved slowing-down power compared with graphite. Critical size, fuel concentration, and breeding ratio were determined for one case, using BeO as moderator.

Since the cost of bismuth as a primary coolant is between $3,000,000 and $4,000,000, the inventory charges are a significant fraction of the total fuel costs. One case was calculated using lead as a coolant in order to compare the increase in inventory charges due to the use of bismuth with the loss in conversion ratio due to the absorptions in the lead.

Basis of nuclear calculations. To obtain comparative results, the following specifications were assumed for all cases:

Average temperature	862°F
Graphite density	1.80 g/cc
Bismuth density	9.83 g/cc
Geometry	Cylinder (H = D)

 

For consistency and ease of comparison, all calculations used equivalent bare reactor dimensions, except the calculation of reflector savings as a function of reflector thickness.

Table 24-6
Summary Of Single-Fluid Nuclear Calculations

Case	Thorium, g/kg Bi, W02	Vs,/Vc	Bare equiv. core size, D = H, ft	Initial conversion ratio	N25,/NBi, X 106
11144	0	0.5	14	0.00	153
11154	0	0.7	14	0.00	134
11164	0	1.0	14	0.00	120
11234	15	0.3	14	0.53	458
11232	15	0.3	10	0.43	621
11244	15	0.5	14	0.608	451
11254	15	0.7	14	0.65	481
11324	30	0.2	14	0.625	774
11325	30	0.2	17	0.666	706
11326	30	0.2	20	0.695	666
11334	30	0.3	14	0.692	772
11335	30	0.3	17	0.733	708
11336	30	0.3	20	0.760	671
11342	30	0.5	10	0.63	1181
11344	30	0.5	14	0.746	870
11345	30	0.5	17	0.788	794
11346	30	0.5	20	0.814	751
11424	50	0.2	14	0.735	1199
11425	50	0.2	17	0.780	1099
11426	50	0.2	20	0.801	1054
11434	50	0.3	14	0.788	1304
11435	50	0.3	17	0.817	1203
11436	50	0.3	20	0.843	1144
11444	50	0.5	14	0.787	1757
11445	50	0.5	17	0.827	1598
11446	50	0.5	20	0.854	1504
11514	80	0.05	14	0.565	2099
11524	80	0.2	14	0.804	1990
11525	80	0.2	17	0.840	1853
11526	80	0.2	20	0.865	1769
11534	80	0.3	14	0.816	2452
11535	80	0.3	17	0.849	2274
11536	80	0.3	20	0.875	2160
11544	80	0.5	14	0.747	4471
11545	80	0.5	17	0.788	3959
11546	80	0.5	20	0.851	3684
11435*	50	0.3	17	0.720	1531
11431†	50	0.3	8	0.680	1223
11433†	50	0.3	12	0.765	1032
11344‡	30	0.5	14	0.880	678
*Lead coolant †BeO moderator ‡U233 fuel

 

 

The resonance integral of the fertile material is a function of the scattering per atom, size of fuel channel, and lattice spacing. The channel size and lattice spacing, however, are not specified; therefore, the lattice resonance parameters are not known. A maximum value of the effective resonance integral is the homogeneous value based on the scattering in the core mixture per atom of fertile material. A minimum value of the resonance integral is the homogeneous value based on scattering in the slurry per fertile atom. For the cases using thorium, a value of Ro2 (effective resonance integral) was chosen between the maximum and minimum values, and the calculated uncertainties are± 20% in the N₂₅/N_Bi ratio and± 3.3% in the conversion ratio.

Results of nuclear calculations. The results of the parametric study are summarized in Table 24-6 for all cases. The critical concentrations and conversion ratios for the cases using thorium as the fertile material are graphed in Figs. 24-21 through 24-25.

The notation used on all graphs have the following definitions:

 

N₂₅/N_Bi = atom ratio of U²³⁵ to bismuth.

W₀₂ = thorium concentration in grams of Th²³²/gBi.

Vs/Vc = volume ratio of slurry to graphite in core.

D = the equivalent bare core diameter in feet.

 

In all cases, the fuel concentration increases with an increase in fertile material, W₀₂ (Fig. 24-24). An increase in Vs/Vc increases the thorium content, reduces the slowing-down power, increases the average energy of the neutron spectrum in the core, and increases the thorium absorptions. As a result of these effects, the critical fuel concentration in the fluid fuel, N2 5/NBi ratio, increases as Vs/Vc increases (Fig. 24-25).

 



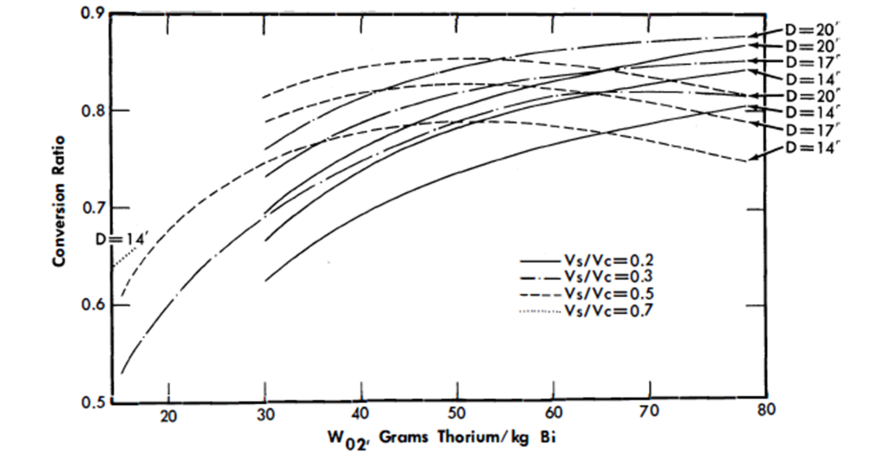
FIG. 24-21. Conversion ratio vs. thorium concentration for a single-fluid LMFR.



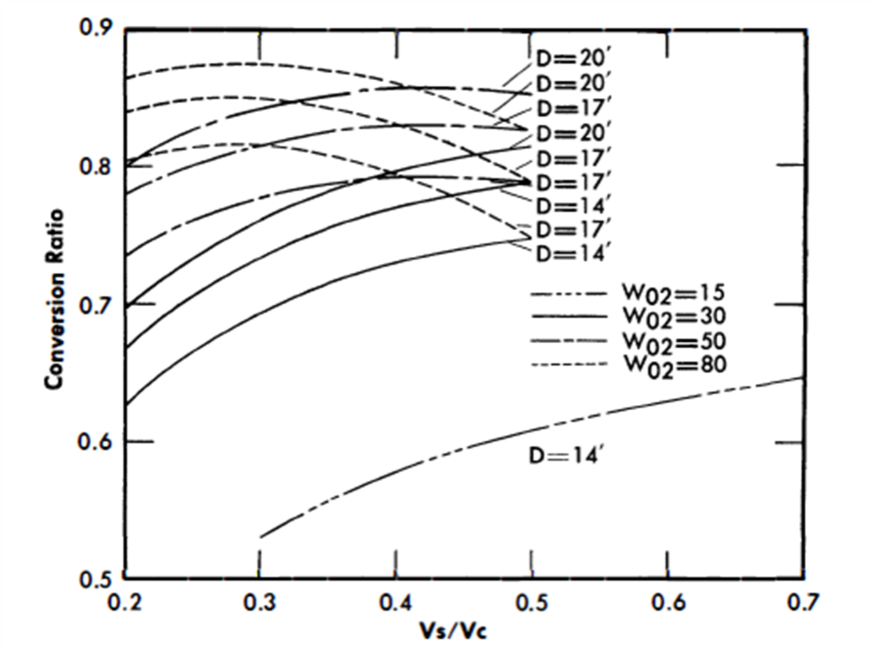
FIG. 24-22. Conversion ratio vs. slurry-to-graphite volume ratio for a single-fluid LMFR.

 



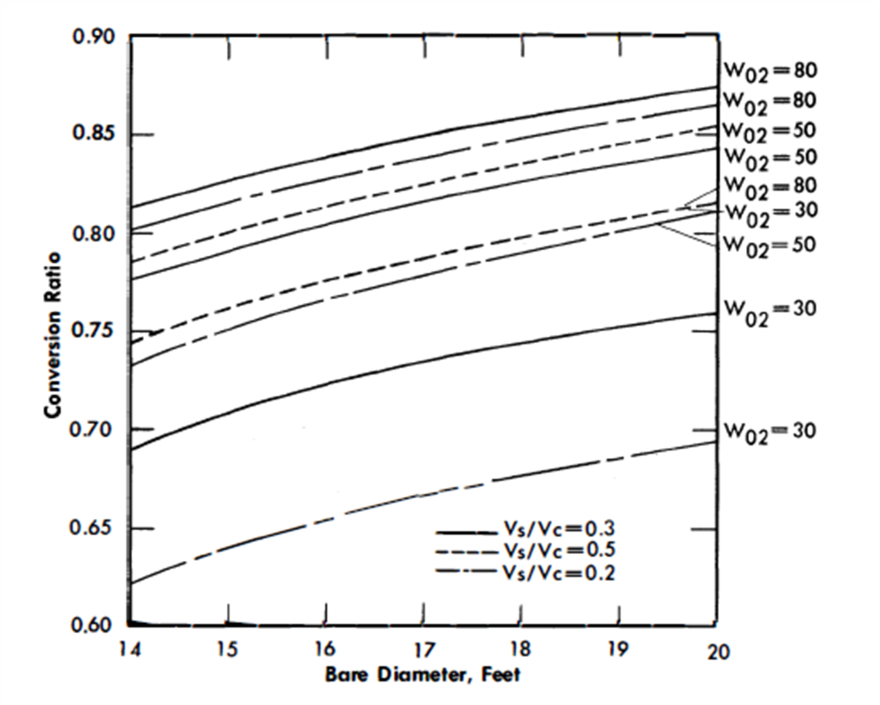
FIG. 24-23. Conversion ratio vs. diameter for a bare single-fluid LMFR.

 

 



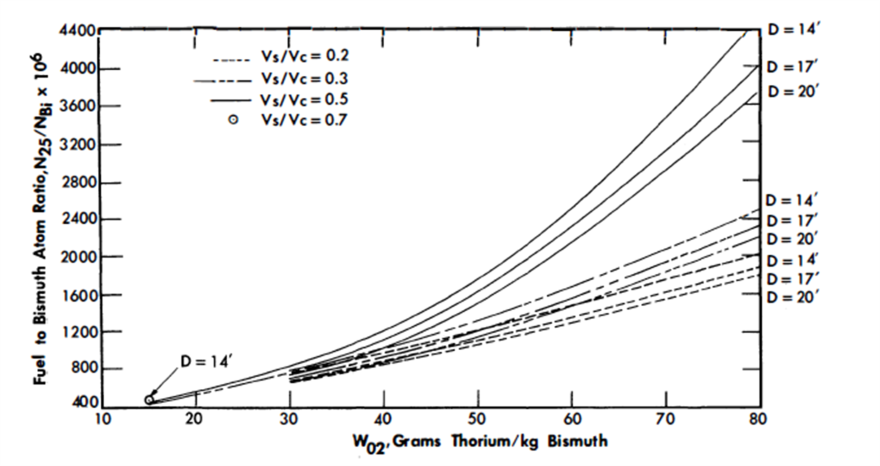
FIG. 24-24. Critical fuel concentration vs. thorium concentration for single-fluid LMFR.

 

 



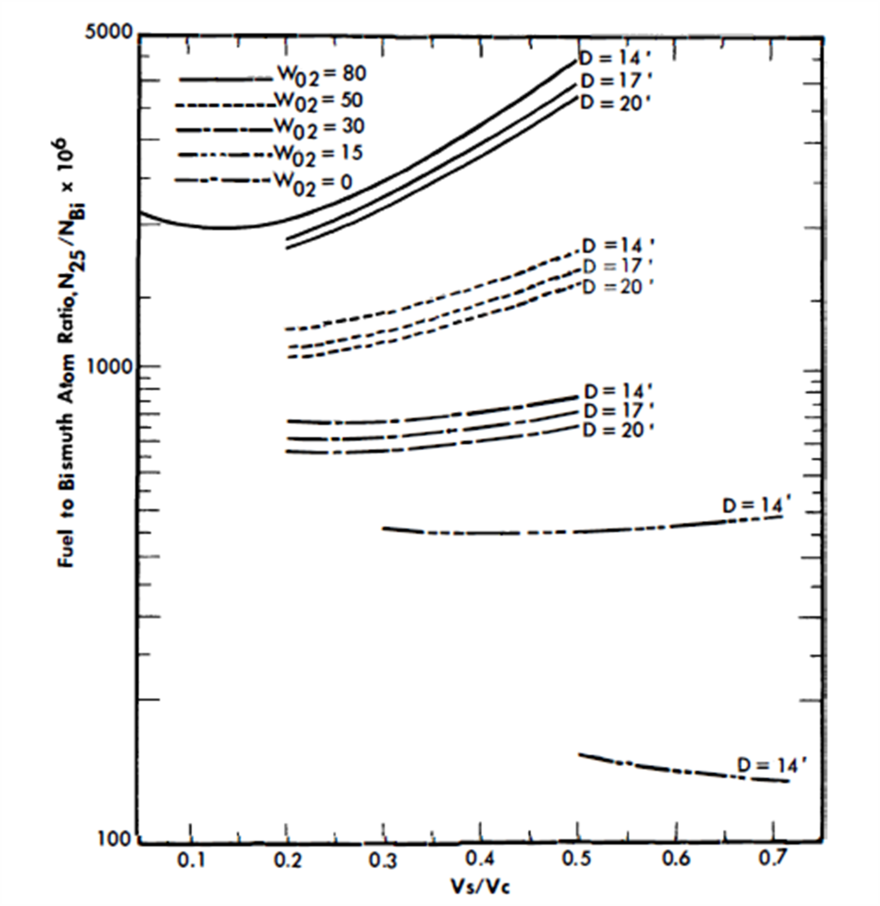
FIG. 24-25. Critical fuel concentration vs. slurry-to-graphite volume ratio for a single-fluid LMFR.

The conversion ratio is highly dependent upon the N₀₂/N₂₅ ratio, the average energy of the neutron spectrum, and the reactor size. Figure 24-21 shows that for larger values of Vs/Vc, the conversion ratio passes through a maximum as thorium concentration increases; however, for smaller values of Vs/Vc, the conversion ratio increases continuously as Vs/Vc increases over the range of interest; i.e., the maximum value of the conversion ratio shifts to higher values of W₀₂ as the Vs/Vc ratio decreases. Likewise, the curves of conversion ratio versus Vs/Vc go through a maximum, with the maximum value occurring at increasingly higher values of Vs/Vc as W0 2 increases (Fig. 24-22).

An increase in core diameter simply reduces the neutron leakage. As a result, the conversion ratio increases as the diameter increases. An increase in D from 14 to 20 ft increases the CR approximately 0.09 (Fig. 24-23).

Case 11435 was recalculated using lead instead of bismuth as the coolant fluid. The conversion ratio decreased by 0.10, and the critical N₂₅/N_Bi ratio increased from 1203 x 10^-6 to 1531 x 10^-6.

Beryllium oxide, BeO, was used as moderator in another variation of Case 11435. This calculation, case 11433, for a diameter of 12 ft, requires an N₂₅/N_Bi ratio of 1032 x 10^-6 and yields the slightly lower conversion ratio of 0.77.

The worth of a pure graphite reflector was calculated for Case 11435. The reflector savings as a function of reflector thickness are shown in Fig. 24-26. The reflector savings are approximately equal to the reflector thicknesses for reflectors less than 2 ft thick.

The values of conversion ratio and N₂₅/N_Bi ratio calculated in this parametric study are for hot, clean reactor conditions, and they are used for comparative purposes only. The effects of fission-product poisons, control rods, and Pa²³³ losses have not been included.

24-4.4 Economic optimization.

The selection of parameters for a reference design must be based upon economics. An economic optimization was accomplished by computing relative energy costs based on those variable costs which depend upon the parameters selected. The costs which are dependent upon the nuclear parameters are (1) bismuth inventory, (2) fuel inventory, (3) fuel burnup, (4) thorium inventory, (5) thorium burnup, (6) reactor core and vessel, and (7) chemical processing costs.

Reactor cost. Since the range of reactor sizes varies from 10 to 20 ft, reactor cost is an important variable. Reactor vessel, graphite, and erection costs have been estimated for several sizes; to these is added $167,000 for three control rods and miscellaneous hardware. Contingency and engineering of 44% were also assumed. A breakdown of these costs is listed in Table 24-7.

 



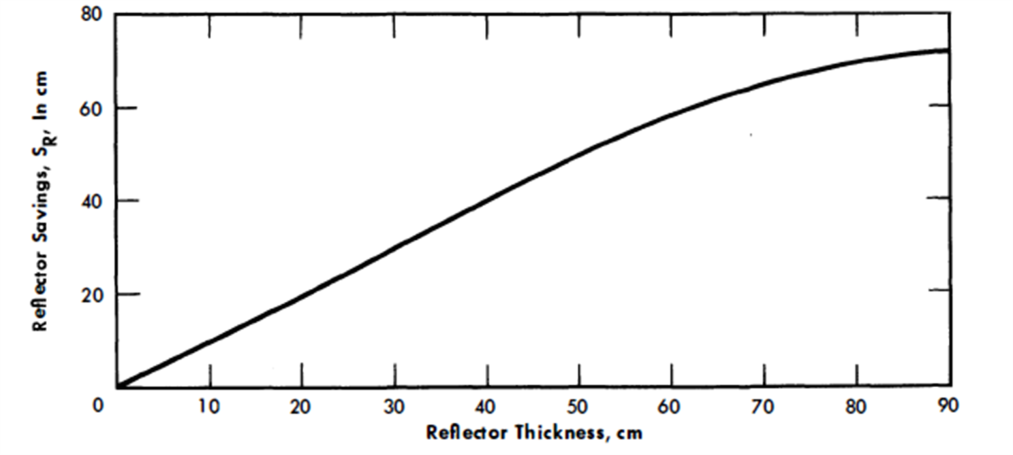
FIG. 24-26. Reflector savings vs. reflector thickness for a single-fluid LMFR. These data are obtained from case 11435, where VsVc = 0.3, W₀₂ 50 g/kg, and D_B= 17.

 

Table 24-7
Estimated Single-Fluid Reactor Cost

 

Size, ft	Reactor	Graphite	Misc.	Erection	Total	Total cost	$/yr
10	160,000	350,000	167,000	24,000	701,000	1,009,440	151,400
14	380,000	970,000	167,000	30,000	1,547,000	2,227,680	334,152
17	570,000	1,700,000	167,000	35,000	2,472,000	3,559,680	533,952
20	900,000	2,800,000	167,000	40,000	3,907,000	5,627,080	843,912

 

Bismuth inventory charges. The bismuth inventory is determined by the primary system volume external to the reactor vessel, the volume of bismuth in the core, the volume of bismuth external to the core but inside the reactor vessel, and the holdup external to the reactor system. The primary system external to the reactor vessel is made up of three heat-exchanger loops containing a total volume of 1640 ft³ The volume of bismuth in the core is

 



 

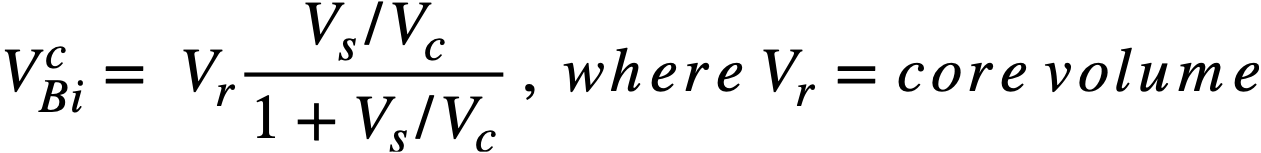
The volume of bismuth external to the core and inside the reactor vessel is tabulated in Table 24-8.

No additional holdup is included to account for temperature expansion during startup, fuel feed system, and other sources of bismuth inventory. The assumption used throughout this study that the volume of bismuth is equal to the volume of slurry accounts for an additional 3 to 10% excess bismuth due to the ThO₂content of the slurry.

 

Table 24-8
Bismuth Inventory In Reactor Vessel External To Core

 

Core, diameter, ft	Bi inventory, ft3
10	550
14	600
17	650
20	700

 

The density of bismuth is taken as 9.83 g/cc, and the price is assumed to be $2.25/lb. Bismuth is a non-depreciating capital investment with a 12% annual amortization rate. The annual bismuth inventory charges may be represented by the equation

 



 

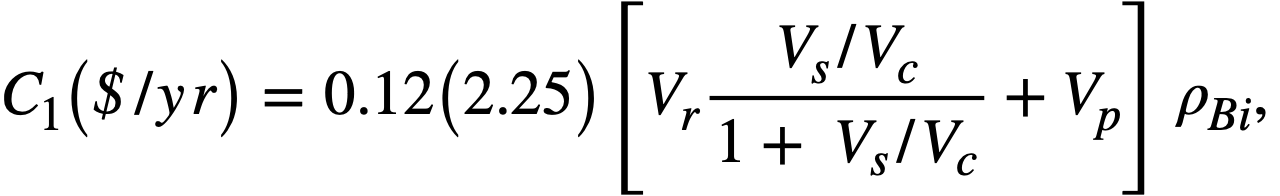
where

V_P= total primary system volume except core, ft³,

ρ_Bi = density of bismuth, lb/ft³.

Fuel inventory charges. The annual lease charges on the U²³⁵ are assumed to be 4%. Treating Pa233 as fuel, the annual fuel inventory charges can be expressed as

 



 

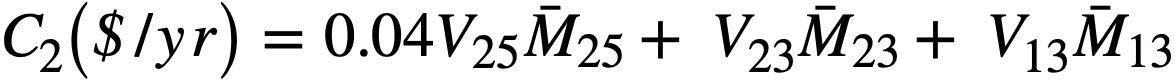
where

V23 = V13 = value of U²³³ as fuel,

V25 = value of U²³⁵ as fuel, $17,760/kg,

 = average mass of element j in entire reactor system during life of plant.

To simplify the work in the absence of information concerning average values of fuel mass, the total mass of fuel was considered to be the hot, clean critical loading at startup. The value of 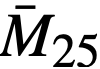 is taken as the initial value with  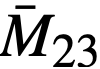and 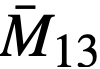 taken as zero.

Fuel burnup costs. Using U²³⁵ as fuel, the yearly burnup costs are

 

 



 

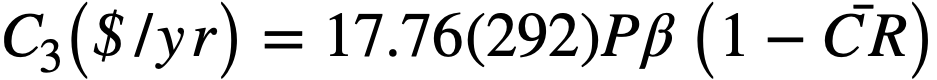
where

P = power, 825 Mw,

β = grams of fuel burned per MwD, 1.25,

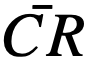 = average conversion ratio.

The initial value of the conversion ratio is used, since only relative costs are needed.

Thorium burnup costs. Thorium is periodically replenished in the reactor to maintain the desired concentration in the slurry. The thorium burnup costs may be expressed as

 



 

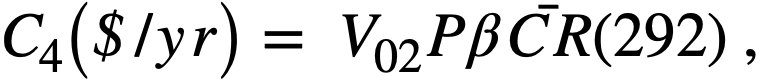
where V₀₂ = value of thorium, $42/kg. These costs are very small, approximately $10,000/yr, and are neglected.

Chemical processing costs. The chemical processing is assumed to use solvent extraction aqueous chemistry in a central processing plant. The irradiated fuel is removed from the reactor on a batch processing cycle. The processing costs are represented by

 



 

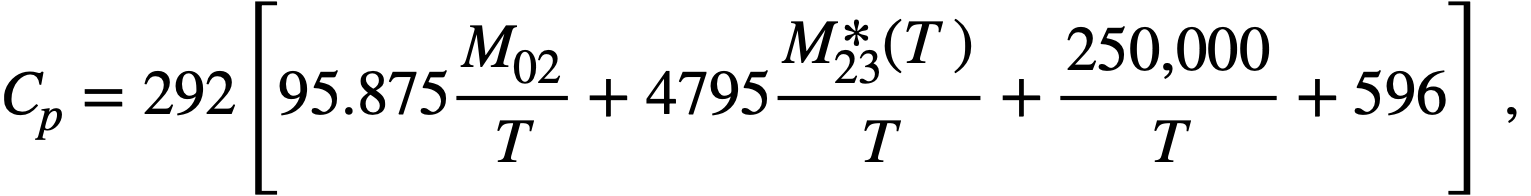
where

 

M₀₂ = total thorium inventory kg,

M₂₃^*(T) = M₂₃ + M₁₃ at time T after loading of fuel charge, kg,

T = chemical processing cycle time, days.

 

Results of economic optimization. Since chemical processing costs are very sensitive to the chemical processing cycle time and the optimum cycle time may vary with reactor design, the relative energy cost of each reactor design was determined neglecting the chemical processing costs. The results of this study are tabulated in Table 24-9 and are shown graphically in Figs. 24-27 and 24-28.

The pure burner, W₀₂ = 0, shows costs more than twice as high as several of concepts (Fig. 24-28). In general, the minimum costs are achieved with thorium loadings corresponding to Wo2 = 20 to 50 g/kg. The most attractive designs do not have the highest values of conversion ratio.

 



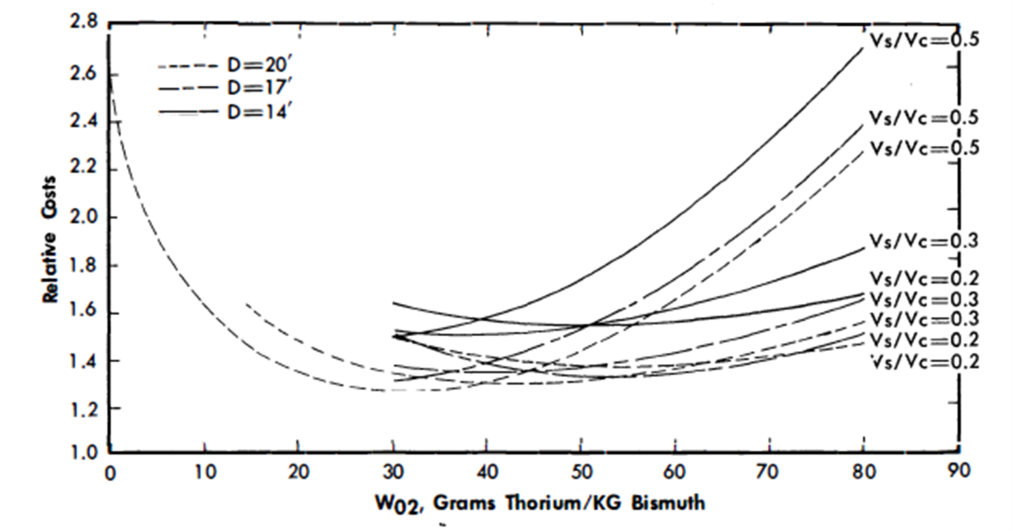
FIG. 24-27. Relative cost vs. thorium concentration for a single-fluid LMFR.

 



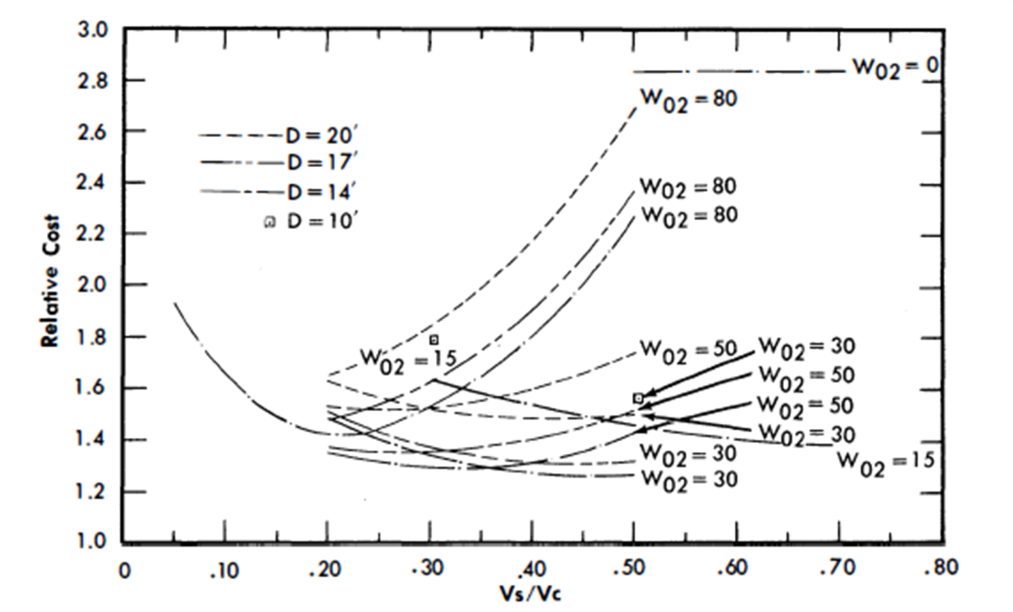
FIG. 24-28. Relative cost vs. slurry-to-graphite volume ratio for a single-fluid LMFR.

 

 

In many cases the additional fuel inventory charges and reactor vessel costs corresponding to higher conversion ratios more than offset the reduction in fuel burnup costs. The economically optimum reactor is neither a burner nor a converter with maximum conversion ratio, but somewhat between these extremes.

Using a cost of 18¢/lb for lead as a coolant, comparisons of lead versus Bi as a coolant were made for Case 11435. The annual fixed charges on lead were only $44,000 compared with $478,000 for bismuth in this case; however, the increased fuel burnup and inventory charges associated with the lead coolant resulted in a net increase of $288,000/yr or 0.14 mills/kwh in the fuel cost. BeO is not feasible as a moderator material for this concept because of its high cost. Fixed charges on the BeO alone add almost a mill/kwh to the fuel cost.

The six most attractive cases were selected and the chemical processing costs computed for several processing cycle times. The total costs tabulated in Table 24-10 are based on a 3000-day cycle time, and other costs are from Table 24-9. Since the aqueous processing costs are dependent upon the total thorium inventory to be processed, chemical processing costs penalize the designs with heavy thorium loadings. In Tables 24-9 and 24-10, the total costs are reduced to mills/kwh by using an electrical power output of 315 Mw with an 80% plant factor.

24-4.5 Selection of a reference design.

Using the data presented in Table 24-10, a design was selected for further study. It is important to realize that when chemical processing costs are included in the comparison of energy costs, there is little difference in the cheapest four or five cases. The relative attractiveness of these cases depends very heavily on the economic ground rules. Even a change in chemical processing cycle may change the relative order of the cases. With the wide range of freedom for choice of nuclear parameters in this concept, the economic optimum can be chosen to correspond to any set of basic assumptions on economics. For example, an increase in fuel price would emphasize higher conversion ratios. The design selected for further study was Case 11344.

Time study. The nuclear performance of the reference design, Case 11344, was determined using a thorium lifetime program written for the digital computer. These calculations provided information concerning the variations of fission-product poisons, breeding ratio, and critical fuel mass as functions of reactor operating time. This information then made possible the choice of an optimum fuel processing cycle and the determination of over-all fuel cost for the operating reactor.

 

Table 24-9
Relative Energy Costs For Single Fluid LMFR

 

Case	Initial conversion ratio	N25/NBi, X 106	W02	Vs,/Vc.	D(bare}, ft	Bismuth inventory, C1 x 10-3, $/yr	Fuel inventory, C2 x 10-3, $/yr	Fuel burnup, C3 x 10-3, $/yr	Thorium inventory, C4 X 10-3, $/yr	Reactor core and vessel, C6 x 10-3, $/yr	CT x 10-3, $/yr	CT, mills/kwh
11144	0	153	0	0.5	14	444	91	5348	0	334	6217	2.82
11154	0	134	0	0.7	14	461	83	5348	0	334	6226	2.82
11164	0	120	0	1.0	14	480	77	5348	0	334	6240	2.83
11232	0.432	621	15	0.3	10	377	310	3039	60	151	3937	1. 78
11234	0.530	458	15	0.3	14	421	256	2516	67	334	3594	1.63
11244	0.609	451	15	0.5	14	444	265	2093	71	334	3207	1.45
11254	0.647	481	15	0.7	14	461	293	1890	73	334	3052	1.38
11324	0.625	774	30	0.2	14	407	411	2007	130	334	3289	1.49
11325	0.666	706	30	0.2	17	451	415	1784	143	534	3327	1. 51
11326	0.695	666	30	0.2	20	512	445	1632	163	844	3596	1.63
11334	0.692	772	30	0.3	14	421	424	1645	134	334	3958	1.34
11335	0.733	708	30	0.3	17	478	442	1427	152	534	3033	1.37
11336	0.760	671	30	0.3	20	560	490	1284	178	844	3356	1.52
11342	0.628	1181	30	0.5	10	421	256	2516	67	151	3411	1.55
11344	0.746	870	30	0.5	14	444	504	1358	141	334	2780	1.26
11345	0.788	794	30	0.5	17	523	541	1136	166	534	2901	1.31
11346	0.814	751	30	0.5	20	478	736	977	254	844	3303	1.50
11424	0.735	1199	50	0.2	14	407	624	1417	216	334	2998	1.36
11425	0.780	1099	50	0.2	17	451	633	1179	239	534	3036	1.38
11426	0.801	1054	50	0.2	20	512	690	1064	272	844	3381	1.53
11434	0.778	1304	50	0.3	14	421	702	1187	223	334	2868	1.30
11435	0.817	1203	50	0.3	17	478	736	977	254	534	2979	1.35
11436	0.843	1144	50	0.3	20	560	820	839	297	844	3359	1.52
11444	0.787	1757	50	0.5	14	444	997	1139	235	334	3149	1.43
11445	0.827	1598	50	0.5	17	523	1068	927	277	534	3330	1.51
11446	0.854	1504	50	0.5	20	637	1226	783	338	843	3828	1.73
11514	0.565	2099	80	0.05	14	381	993	2325	323	334	4256	1. 93
11524	0.804	1990	80	0.2	14	407	1007	1051	345	334	3144	1.42
11525	0.840	1853	80	0.2	17	451	1038	857	382	534	3263	1.48
11526	0.865	1769	80	0.2	20	512	1126	724	434	844	3640	1.65
11534	0.816	2452	80	0.3	14	421	1284	986	357	334	3382	1.53
11535	0.850	2274	80	0.3	17	478	1352	803	406	534	3574	1.62
11536	0.875	2160	80	0.3	20	560	1504	671	475	844	4053	1.84
11544	0.747	4471	80	0.5	14	444	2462	1356	376	334	4972	2.25
11545	0.788	3959	80	0.5	17	523	2569	1132	444	534	5201	2.36
11546	0.815	3684	80	0.5	20	637	2912	990	541	844	5924	2.68

Table 24-10
Relative Fuel Costs Including Chemical Processing Single Fluid LMFR

 

Assumed Processing Cycle = 3000 Days

 

Case No.	W02	Initial CR	Vs/Vc	D(bare), ft	M02 kg	M25, kg	Chemical processing cost, CP x 10-3 /yr	Other cost, CT x 10-3/yr	Total costs, 10-3/yr	Total costs, mills/kwh
		-
11254	15	0.65	0.7	14	11,640	413	504	3052	3556	1. 61
11334	30	0.69	0.3	14	21,268	597	681	2958	3639	1.65
11344	30	0.75	0.5	14	22,407	709	745	2780	3525	1.60
11345	30	0.79	0.5	17	26,407	762	808	2901	3709	1.68
11434	50	0.78	0.3	14	35,447	989	1000	2868	3868	1. 75
11435	50	0.82	0.3	17	40,275	1036	1068	2979	4047	1.83

 

 

 

Basis of time study. The reference design calculations used U²³³ as fuel for both the initial charge and feed material. Since the contemplated construction date for an LMFR is 10 yr in the future, the assumption that U²³³ fuel will be available seems reasonable, and data based on U²³³ allows comparison with previous work [2].

The reference design on which the time studies were based has a graphite side reflector 1.5 ft thick, an active core diameter of 11 ft, and a core height of 14 ft. The average core temperature is 900°F. The nuclear constants used in the two-group criticality and isotope buildup calculations were determined by using a 40-group spectral code.

The neutron poisons due to fission products and higher uranium isotopes were calculated using the data by W. L. Robba et al. [4]. The xenon poisoning (absorptions in Xe¹³⁵ to absorptions in fuel) was held at 0.01 throughout life, and Sm149 was allowed to reach steady state. The other fission-product poisoning corresponds to poison data labeled “less Xe and Sm with high cross sections except low Zr⁹³.” Due to lack of information, no resonance absorption by the fission products was considered. The neutron flux averaged over the entire primary system volume was used in all isotope and poison buildup computation, since this is a circulating fuel reactor.

Fuel was added at frequent time intervals to maintain kerr 1.01 (assuming 1% rod holddown). Thorium was added to the core with the fuel to maintain a constant thorium loading.

Results of time study. The study was carried to 2000 days of full-power operation. The mass of U²³³ fuel and the buildup of Pa²³³ are shown in Fig. 24-29, and the buildup of fission product poisons (other than Xe¹³⁵ and Sm¹⁴⁹) along with breeding ratio are graphed in Fig. 24-30. The fission­product poisons vary in an almost linear manner for burnups corresponding to 2000 to 6000 days. Other calculations have indicated that extrapolations (represented by dashed lines on Figs. 24-29 and 24-,--30) to 5840 days, the expected life of the plant, are reasonable.

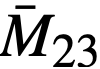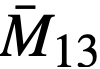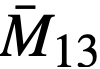The quantities necessary to evaluate the chemical processing costs for various processing cycles are average values of fuel mass and breeding ratio (, , , and ). The average value of , is approximately the steady-state value; 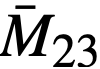, and  are shown in Figs. 24-29 and 24-30.

Selection of chemical processing cycle. The fuel costs which are dependent upon the chemical processing cycle are fuel inventory, fuel burnup, and processing charges. These charges were computed using formulas similar to those described in Article 24-4.4 but using data appropriate to U²³³ fuel. Equations giving costs in dollars per full power day are

 

 

Fuel inventory: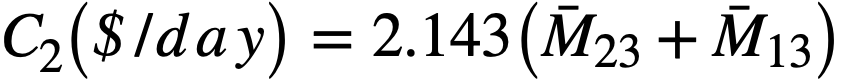 

Fuel burnup: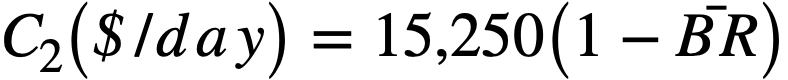 

Chemical processing:



 

The results of these calculations are tabulated in Table 24-11 and graphed in Fig. 24-31. This analysis indicates an economic optimum processing cycle of approximately 4000 full-power days. However, only a small penalty of slightly more than $200/day (less than 0.03 mills/kwh) is incurred by operating the reactor for its complete life (5840 full-power days) before sending the fuel to a chemical separations plant.

 



FIG. 24-29. Mass of U²³³ vs. reactor operating time for a single-fluid reactor operating at 825 Mw.

 



FIG. 24-30. Neutron losses vs. reactor operating time for a single-fluid reactor operating at 825 Mw.

 



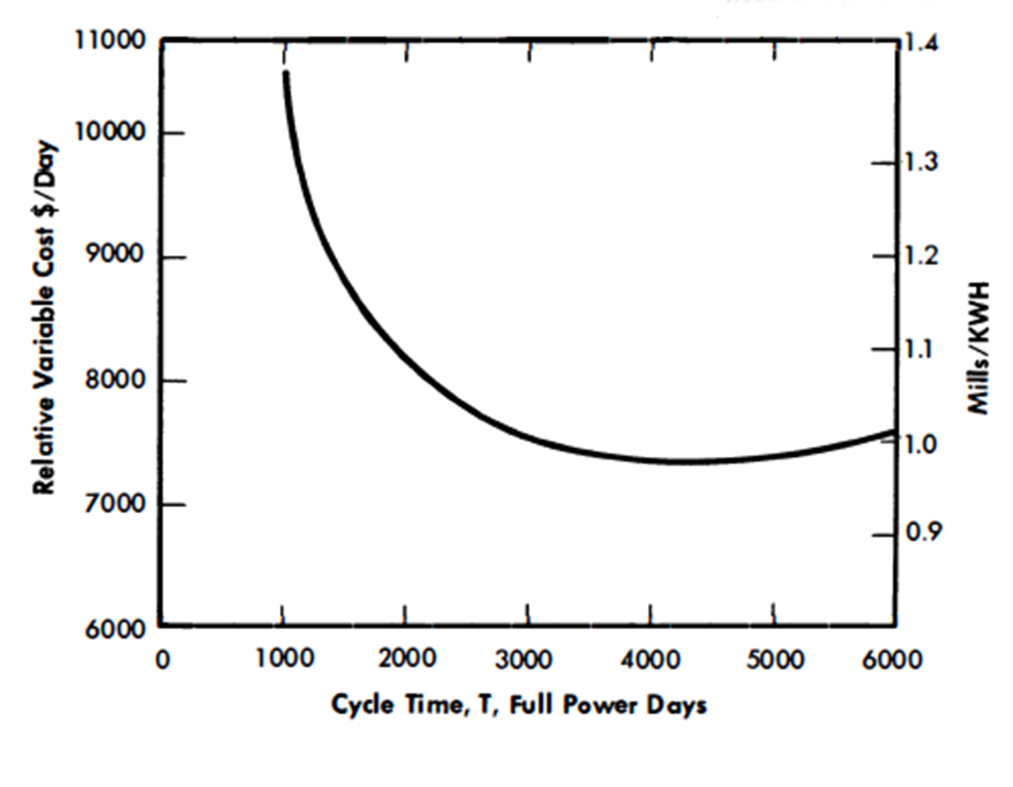
FIG. 24-31. Variable fuel cost for 825-Mw single fluid LMFR vs. chemical processing cycle (aqueous batch process).

 

 

Table 24-11
Variable Fuel Cost For An 825-Mw Lmfr

For various processing cycles

 

 

Chern. proc. cycle, days	M23(T)	M13(T)	M23(T)	M13(T)	M*23(T)	BR(T)	Fuel inventory charges, $/day	Fuel burnup costs, $/day	Chern. proc. costs, $/day	Total variable cost, $/day
6000	780	30	730	30	760	0.722	1690	4240	1,627	7,557
4000	725	30	675	30	705	0.755	1510	3738	2,101	7,349
2000	671	30	623	30	653	0.787	1400	3230	3,575	8,105
1000	635	30	595	30	625	0.807	1340	2943	6,184	10,470
500	603	30	578	30	608	0.820	1303	2746	16,467	15,500

 



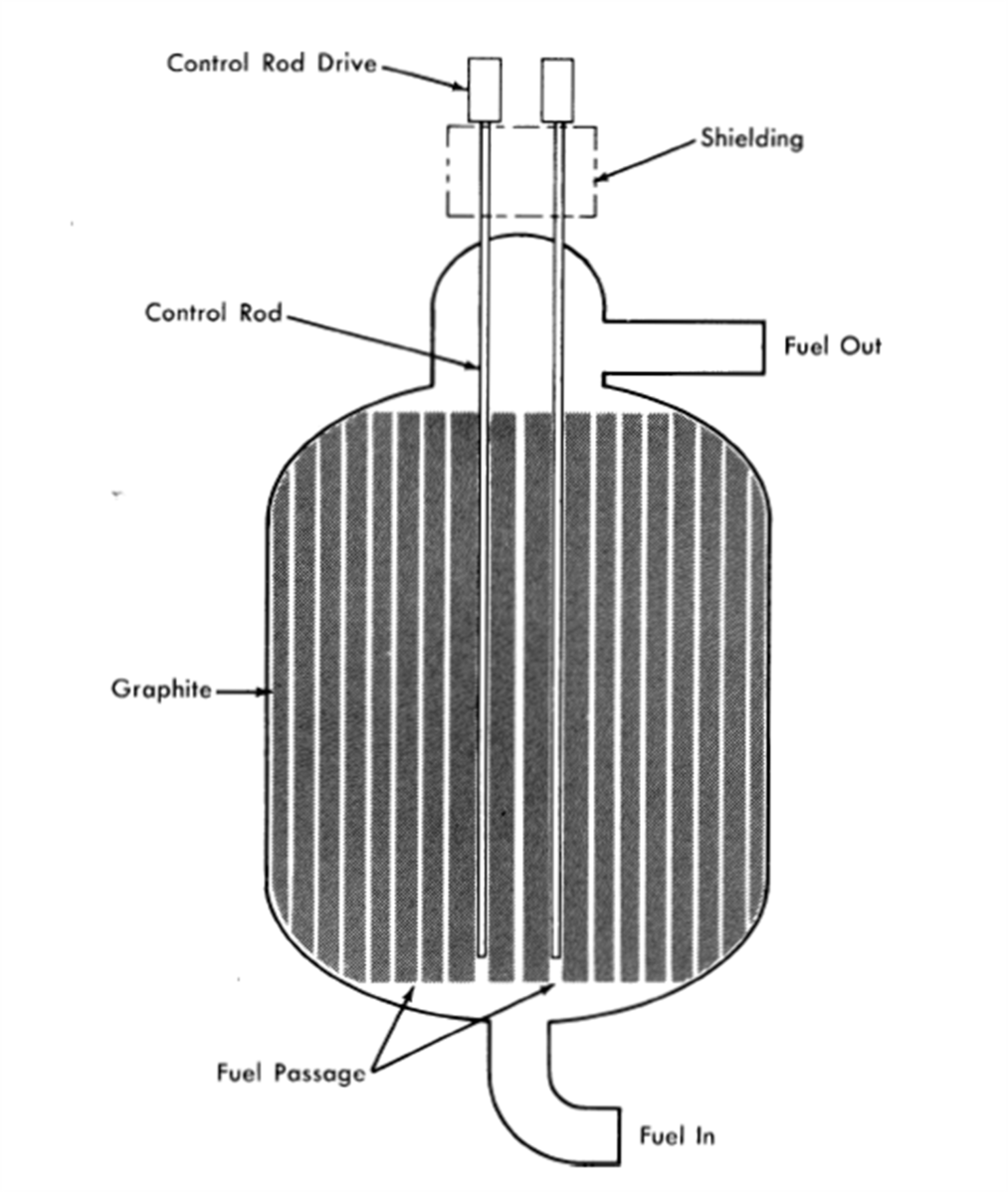
FIG. 24-32. Single-region, externally cooled liquid metal fuel reactor.

 

Specifications of reference design. The single-region reactor design is illustrated in Fig. 24-32. The core is constructed of large blocks of high density reimpregnated graphite, with 1.5- to 2.0-in.-diameter axial holes for the passage of fuel slurry. The graphite is supported by a number of compensated molybdenum rods and a bottom support plate. Provision is made for three or four liquid metal control rods, if experience indicates they are necessary.

The reactor vessel is constructed of 2¼% Cr-1% Mo steel, 2¼ inches thick, designed for a temperature of 1150°F and maximum pressure of 120 psi. Three 28-in.-diameter pipes carry the fluid into the reactor at the bottom and leave at the top. The entire reactor vessel is doubly contained by a relatively thin-walled containment vessel. A drain line to the fuel dump tanks is also provided. The free space above the reactor core is used as the degasser to remove volatile fission products. The reference core design has the following specifications:

 

Power:
Thermal power	825Mw
Net electrical power	315,000 kw
Station efficiency	38.2%
Materials:
Fuel	U233
Fertile material	Thorium
Moderator	High-density graphite
Reflector	High-density graphite
Coolant	UO2-ThO2-Bi Slurry
Coolant-to-moderator ratio, Vs./Vc	0.5
Thorium concentration, W02	30 g/kg Bi
Geometry:
Core radius	5.5 ft
Core height	14.0 ft
Reflector thickness	1.5 ft
Number of primary coolant loops	3
Fuel-slurry volume:
Coolant loops 1640 ft3
Reactor core 443
Reactor vessel 600
Total	2683 ft3
Chemical processing cycle	4000 days
Nuclear data:
	Startup	4000 days	Average
Mass U233	546 kg	725 kg	675 kg
Mass Pa233	0	30	30
Mass 233	546	755	705
Initial average core thermal flux			3 X 1014
Breeding ratio	0.87	0.725	0.75
Poison fraction	0	0.216
Mass of bismuth	1,646,000 lb
Mass of thorium	22,400 kg

24-5. Economics

Economic considerations were essential to the optimization studies required to establish the reference designs presented in Sections 24-2 and 24-4. An important objective of this study is the economic comparison of energy costs for the single-fluid and the two-fluid externally cooled LMFR. A brief summary of energy costs for the optimum design in each concept is presented in Table 24-12.

 

Table 24-12
Energy Cost (80% Plant Factor)

	Mills/kwh
Single-fluid LMFR	Two-fluid LMFR
Fixed charges on total capital investment	4.09	4.31
Nuclear fuel and inventory costs	1.24	1.41
Maintenance	1.18	1.05
Operation	0.38	0.38
Interest on working capital	0.04	0.04
Total energy cost, mills/kwh	6.9	7.2

 

24-5.1 Fixed charges on capital investment.

Direct construction costs. The estimated costs of equipment, installation of equipment, and construction are based on the plant layouts for the two reference designs evaluated in this study. Construction and erection costs of all items, as well as direct materials costs for those components manufactured by the Babcock & Wilcox Company, were developed by B&W estimators. Delivered costs of equipment supplied by manufacturers other than B&W were taken from vendors' quotations.

A summary of direct construction costs for each reference design is tabulated in Tables 24-13 and 24-14.

Total capital investment. The total capital investments are summarized according to account numbers in Tables 24-15 and 24-16.

24-5.2 Maintenance and operation.

In computing energy costs, the fixed charges on maintenance equipment and spare parts are included in the maintenance costs, while fixed charges on buildings used for maintenance are included in fixed charges on capital investment.

 

Table 24-13
Summary Of Direct Construction Costs For Single-Fluid Lmfr

 

Account no.		Direct labor	Direct materials	Direct construction cost
310	Land and land rights
	Direct site		$500,000	$500,000
311	Structures and improvements
	Improvements and miscellaneous structures	$78,000	126,000	204,000
	Nuclear steam generator building	2,981,000	3,535,000	6,516,000
	Accessory buildings	51,000	84,000	135,000
	Total	3,110,000	3,745,000	6,855,000
312	Nuclear Steam Generator and Chemical Plant Equipment
	Reactor	60,000	2,028,000	2,088,000
	Primary and blanket system	309,000	1,470,000	1,779,000
	Blanket system			3,057,000
	Intermediate system	279,000	2,778,000
	Steam system	125,000	1,629,000	1,754,000
	Primary inert gas system (He)	5,000	22,000	27,000
	Intermediate gas system (N 2)	6,000	24,000	30,000
	Reactor heating and cooling system	21,000	84,000	105,000
	Dump tank heating and cooling systems	100,000	149,000	249,000
	Primary system capsule area ventilating system	1,000	2,000	3,000
	Primary system reactor cell cooling system	3,000	3,000	6,000
	Shield cooling system	18,000	21,000	39,000
	Water cooling system	3,000	7,000	10,000
	Off-gas system	20,000	50,000	70,000
	Feed-water heating system	286,000	2,541,000	2,827,000
	Instrumentation and controls	599;000	1,236,000	1,835,000
	Spare parts	110,000	2,666,000	2,776,000
	Miscellaneous equipment	315,000	588,000	903,000
	Inventories		4,680,000	4,680,000
	Chemical plant equipment	156,000	403,000	559,000
	Total	2,416,000	20,381,000	22,797,000
314	Turbine Generator Equipment
	Turbine and condensing	828,000	13,382,000	14,210,000
315	Accessory electrical equipment	400,000	2,484,000	2,884,000
316	Miscellaneous power plant equipment
	Transmission structures	50,000	130,000	180,000
	Maintenance equipment	172,000	2,921,000	3,093,000
	Total	222,000	3,051,000	3,273,000
342-373	Station equipment	189,000	1,246,000	1,435,000
	Total Direct Construction Cost			$ 51,954,000

 

 

Table 24-14

Summary Of Direct Construction Costs ·For Two-Fluid LMFR

 

Account no.		Direct labor	Direct materials	Direct cost construction
310	Land and land rights
	Direct site		$500,000	$500,000
311	Structures and improvements
	Improvements and miscellaneous structures	$78,000	126,000	204,000
	Nuclear steam generator building	3,184,000	4,035,000	7,219,000
	Accessory buildings	51,000	84,000	135,000
	Total	3,313,000	4,245,000	7,558,000
312	Steam generator and chemical plant equipment
	Reactor	50,000	1,531,000	1,581,000
	Primary and blanket system	436,000	1,788,000	2,224,000
	Intermediate system	295,000	3,365,000	3,660,000
	Steam system	250,000	1,949,000	2,199,000
	Primary inert gas system (He)	8,000	34,000	42,000
	Intermediate gas system (N 2)	8,000	40,000	48,000
	Reactor heating and cooling system	21,000	84,000	105,000
	Dump tank heating and. cooling systems	100 , 000	149,000	249,000
	Primary system capsule area ventilating system	1,000	2,000	3,000
	Primary system reactor cell cooling system	3,000	3,000	6,000
	Shield cooling system	18,000	21,000	39,000
	Water cooling system	3,000	7,000	10,000
	Off-gas system	20,000	50,000	70,000
	Feed-water heating system	290,000	2,546,000	2,836,000
	Instrumentation and controls	765,000	1,547,000	2,312,000
	Spare parts	72,000	2,000,000	2,072,000
	Miscellaneous equipment and inventories	273,000	564,000	837,000
	Inventories		4,289,000	4,289,000
	Chemical plant equipment	540,000	1,461,000	2,001,000
	Total	3,153,000	21,430,000	24,583,000
314	Turbine generator equipment
	Turbine and condensers	828,000	13,382,000	14,210,000
315	Accessory electrical equipment	401,000	2,503,000	2,904,000
316	Miscellaneous power plant equipment
	Transmission structure	50,000	130,000	180,000
	Maintenance equipment	173,000	2,274,000	2,447,000
	Total	223,000	2,404,000	2,627,000
342-343	Station equipment	189,000	1,246,000	1,435,000
	Total Direct Construction Cost	$8,107,000	$45,710,000	$53,817,000

 

 

Table 24-15

Summary Of Capital Investment For Single-Fluid Lmfr

 

Account no.		Direct construction costs	Contingency	Miscellaneous charges *	Total capital investment
310	Land and land rights	$500,000	$0	$0	$500,000
311	Structures and tmprovements
	Nuclear and turbogenerator plant	6,697,000	1,479,000	3,158,000	11,334,000
	Chemical plant	158,000	34,000	71,000	263,000
312	Nuclear steam generating and chemical
	plant equipment
	Nuclear plant equipment	14,782,000	2,733,000	3,436,000	20,951,000
	Chemical plant equipment	559,000	112,000	188,000	859,000
	Spare parts	2,776,000	479,000	418,000	3,673,000
	Inventories	4,680,000	°	°	4,680,000
314	Turbine generator equipment	14,210,000	2,483,000	2,346,000	19,039,000
315	Accessory electrical equipment	2,884,000	531,000	655,000	4,070,000
316	Miscellaneous power plant equipment
	Transmission structures	180,000	36,000	61,000	277,000
	Maintenance equipment	3,093,000	539,000	505,000	4,137,000
342-343	Station equipment	1,435,000	263,000	318,000	2,016,000
	Total	$51,954,000	$8,689,000	$11,156,000	$71,799,000

*Includes indirect construction cost, interest, and engineering charges.

 

 

Table 24-16
Summary Of Capital Investment For Two-Fluid LMFR

 

Account		Direct			Miscellaneous	Total capital
	construction		Contingency
no.		costs			charges	.investrnent
310	Land and land rights	$500,000		$0	$0	$500,000
311	Structures and improvements
	Nuclear and turbogenerator plant	7,010,000		1,538,000	3,223,000	11,771,000
	Chemical plant	548,000		119,000	247,000	914,000
312	Nuclear steam generating and chemical
	plant equipment
	Nuclear plant equipment	16,221,000		2,930,000	3,915,000	23,066,000
	Chemical plant equipment	2,001,000		401,000	658,000	3,060,000
	Spare parts	2,072,000		360,000	301,000	2,733,000
	Inventories	4,289,000				4,289,000
314	Turbine generator equipment	14,210,000		2,483,000	2,346,000	19,039,000
315	Accessory electrical equipment	2,904,000		534,000	659,000	4,097,000
316	Miscellaneous power plant equipment
	Transmission structures	180,000		36,000	61,000	277,000
	Maintenance equipment	2,447,000		432,000	427,000	3,306,000
342-343	Station equipment	1,435,000		263,000	318,000	2,016,000
	Total	$53,817,000		$9,096,000	$12,155,000	$75,068,000

 

 

Table 24-17 Summary Of Fuel Costs

315 Mw (Elec.); Plant Factor = 80%

 

Item	Capital investment	Annual cost, $/yr	Energy cost, mills/kwh
Single-fluid	Two-fluid	Single-fluid	Two-fluid	Single-fluid	Two-fluid
Bismuth inventory	$3,704,000	$3,090,000	$444,000	$371,000	0.201	0.168
Fuel inventory				455,000	396,000	0.206	0.179
FUel burnup				1,091,000	627,000	0.494	0.284
Thorium inventory	941,000	1,171,000		141,000	176,000	0.064	0.080
Thorium burnup				9,000	10,000	0.004	0.005
Chemical processing:
Offsite processing				144,000		0.063
Buildings	263,000	941,000		35,000	127,000	0.016	0.058
Equipment	859,000	3,060,000		116,000	744,000	0.053	0.337
Operating costs				18,000	530,000	0.008	0.240
Shipping charges				6,000		0.002
Thorium inventory				13,000		0.006
Fuel inventory				44,000		0.020
Fuel depreciation				222,000	124,000	0.101	0.056
Total	$5,767,000	$8,262,000		$2,744,000	$3,105,000	l.24	1.4l

 

Table 24-18
U nit Energy Costs

Item	Cost, mills/kwh
Single-fluid	Two-fluid
Land and land rights	0.03	0.03
Structures and improvements (less chemical processing facilities)	0.69	0.72
Equipment (less maintenance equipment and spares) :
Reactor vessel and internals	0.19	0.14
Primary and blanket system	0.21	0.27
Intermediate system	0.36	0.44
Feedwater heating system	0.26	0.27
Instrumentation and controls	0.29	0.36
Miscellaneous equipment and Na inventory	0.11	0.10
Auxiliary systems	0.22	0.27
Station equipment	0.14	0.14
Accessory electric equipment	0.28	0.28
Turbine generator equipment	1.29	1.29
Miscellaneous power plant equipment	0.02	0.02
Fuel costs (includes chemical processing facilities)	1.24	1.41
Plant operation	0.38	0.38
Maintenance (includes maintenance equipment and spares)	1.18	1.05
Interest on working capital	0.04	0.04
Total	6.93	7.21

 

24-5.3 Fuel costs.

The fuel costs as presented in this report include (1) bismuth inventory, (2) fuel inventory, (3) fuel burnup, (4) thorium inventory, (5) thorium burnup, and (6) chemical processing. Sodium inventory is not included, since it is used as coolant fluid for the intermediate system and does not contain fuel. Fuel costs are summarized in Table 24-17.

24-5.4 Summary of energy costs.

The energy costs in mills/kwh, based upon an electric output of 315,000 kw and a plant factor of 80%, are tabulated in Table 24-18 for various categories.

 

 

References

1. Babcock & Wilcox Co., 1958. USAEC Report BAW-1046.

2. Babcock & Wilcox Co., Liquid Metal Fuel Reactor; Technical Feasibility Report, USAEC Report BAW-2(Del.), June 30, 1955.

3. Babcock & Wilcox Co., 1958. USAEC Report BAW-1048.

4. W. L. Robba et al., Fission-product Buildup in Long-burning Thermal Reactors, Nucleonics 13(12), 30-33 (1955).

5. Babcock & Wilcox Co., A Review and Evaluation of Maintenance Concepts for Liquid Metal Fuel Reactors, USAEC Report BAW-1047, March 1958.